Relative permeability

In physics, in particular in magnetostatics, the relative permeability is an intrinsic property of a magnetic material. It is usually denoted by μ_r. For simple magnetic materials, using SI units, μ_r is related to the proportionality constant between the magnetic flux density B and the magnetic field H, namely B = μ_r μ₀ H, where μ₀ is the magnetic constant. The relative permeability describes the ease by which a magnetic medium may be magnetized.

A related quantity is the magnetic susceptibility, denoted by χ_m, related to the relative permeability in SI units by:

$\mu _{r}=1+\chi _{m}\ .\ \mathrm {SI\ units} \ .$

The relative permittivity μ_r may be greater than or less than 1. For simple media, if μ_r < 1 (χ_m negative), the medium is termed diamagnetic; if μ_r > 1 (χ_m positive) paramagnetic. Only classical vacuum has μ_r = 1 (exact). A typical diamagnetic susceptibility is about −10⁻⁵, while a typical paramagnetic susceptibility is about 10⁻⁴.

Relation to magnetization

The magnetic flux density B is related to the magnetization of a magnetic medium M as:

$\mathbf {B} =\mu _{0}\mathbf {H+M} \ .$

In a simple magnetic material, the magnetization induced by H is simply proportional to the field H, and is given in terms of the medium's magnetic susceptibility as:

$\mathbf {M} =\chi _{m}\mu _{0}\mathbf {H} \ .$

Consequently, the magnetic flux density in such a magnetic medium is simply:

$\mathbf {B} =(1+\chi _{m})\mu _{0}\mathbf {H} =\mu _{r}\mu _{0}\mathbf {H} =\mu \mathbf {H} \ .$

Here μ is referred to as the magnetic permeability.

In implementing these relations, it should be noted that the use of a constant as the relative permeability of a substance is an approximation, even for quantum vacuum. A more complete representation recognizes that all media exhibit departures from this approximation, in particular, a dependence on field strength, a dependence upon the rate of variation of the field in both time and space, and a dependence upon the direction of the field. In many materials these dependencies are slight; in others, like ferromagnets, they are pronounced.

Relation to forces

See also Ampère's equation.

Similar to Coulomb's law describing the electric force between electric charges, the magnetic force between two magnetic poles of pole strength given by p₁ and p₂ and separated by distance r in a medium of relative permeability μ_r has magnitude F:

$F={\frac {\mu _{r}\mu _{0}}{4\pi }}\ {\frac {p_{1}p_{2}}{r^{2}}}\ ,$

and is directed along the line joining the poles. It is attractive if the poles are opposite in nature (one north pole and one south pole) and repulsive if they are the same.

It happens, however, that magnetic poles always occur as opposite pairs, so the force law between single poles cannot be observed directly. However, magnetic dipoles do occur in nature and can be directly observed. A planar wire loop conducting a steady electric current resembles a magnetic dipole at distances large compared to the dimensions of the loop.

The magnetic force between two charges moving at constant velocity (or for accelerating charges with velocities well below the speed of light) in a medium of relative permeability μ_r is provided by the Lorentz force acting upon one charge via the magnetic flux density generated by the other as predicted by the Biot-Savart law. Let the charges be q₁, q₂, their separation be r₁₂, and their constant velocities be v₁, v₂. The magnetic flux density B generated by charge 2 at a position P in classical vacuum is:

$\mathbf {B} ={\frac {\mu _{0}}{4\pi }}\ {\frac {q_{2}\mathbf {v_{2}\ \times \ {\hat {u}}} }{r^{2}}}\ ,$

where û is a unit vector pointing from the charge to the field evaluation point P, and r is the distance between the charge and the field point. The corresponding magnetic field H is:

$\mathbf {H} ={\frac {1}{4\pi }}\ {\frac {q_{2}\mathbf {v_{2}\ \times \ {\hat {u}}} }{r^{2}}}\ .$

If now the charges are placed in a magnetic medium, the field H induces a magnetization M in the medium, and within the assumption of a simple linear magnetic material M = χ_mμ₀ H. Thus, the medium changes B = μ₀H to B = μ₀(1+χ_m)H and the flux density in the medium becomes:

$\mathbf {B} ={\frac {\mu _{r}\mu _{0}}{4\pi }}\ {\frac {q_{2}\mathbf {v_{2}\ \times \ {\hat {u}}} }{r^{2}}}\ .$

Then the magnetic force upon charge 1 located at P₁ due to charge 2 according to the Lorentz law is:

${\begin{aligned}\mathbf {F_{21}} &=q_{1}\mathbf {v_{1}\ \times \ B} (P_{1})\\&={\frac {\mu _{r}\mu _{0}}{4\pi }}\ {\frac {q_{1}q_{2}}{r_{12}^{2}}}\left(\mathbf {v_{1}\ \times \ } (\mathbf {v_{2}\ \times \ {\hat {u}}_{12}} )\right)\ ,\\\end{aligned}}$

where û₁₂ is a unit vector pointing from charge 2 to charge 1 and r₁₂ is their separation. This expression can be expressed in terms of the normal scalar products of the vectors using the baccab formula for the triple vector product:

$\mathbf {F_{21}} ={\frac {\mu _{r}\mu _{0}}{4\pi }}\ {\frac {q_{1}q_{2}}{r_{12}^{2}}}\left((\mathbf {v_{1}\cdot {\hat {u}}_{12}} )\mathbf {v_{2}} -(\mathbf {v_{1}\cdot v_{2}} )\mathbf {{\hat {u}}_{12}} \right)\ .$

This expression does not satisfy Newton's law of action and reaction, which would require the force on charge 1 due to charge 2 be equal and opposite to the force on particle 2 due to charge 1. But it does not have to, because it does not describe an instantaneous action-at-a-distance, but rather, a force mediated by propagation of the electromagnetic field.

(PD) Image: John R. Brews
Infinitesimal current elements in two closed current-carrying loops

This relation is closely connected to the force between two current elements, as described by Grassmann's adaptation of Ampère's equation, described next.

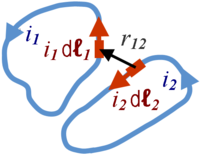
The force exerted between infinitesimal current elements from two loops carrying steady currents i_i and i_j and immersed in a magnetic medium with relative permeability μ_r is changed by a factor μ_r. The infinitesimal current elements have vector components i₁dℓ₁ and i₂dℓ₂ where the incremental lengths are directed along the wire at the location of the element, and pointing in the direction of the current. Grassmann's law for the force upon current element 1 exerted by current element 2 is:

$d^{2}\mathbf {F_{21}} =-i_{1}i_{2}{\frac {\mu _{r}\mu _{0}}{4\pi }}{\frac {1}{r_{12}^{2}}}\left(\ (d{\boldsymbol {\ell _{1}\cdot }}d{\boldsymbol {\ell _{2}}})\mathbf {{\hat {u}}_{12}} -(\mathbf {{\hat {u}}_{12}\cdot } d{\boldsymbol {\ell _{1}}})d{\boldsymbol {\ell _{2}}}\right)\ ,$

as is readily derived from the Biot-Savart law, and is consistent with space-time symmetry. Here û₁₂ is a unit vector pointing from element 2 to element 1 along the line joining them, and r₁₂ the length of this line. The force element is second order because it is a product of two infinitesimals.

Background

Maxwell reduced electrodynamics to the knowledge of charge and current distributions, and introduced the notion of material properties (constitutive relations) relating magnetic flux density B to magnetic field H and electric displacement D to electric field E.