This is an experimental draft. For a brief description of the project and motivations click here.
Complex numbers are defined as ordered pairs of reals:

Such pairs can be added and multiplied as follows
- addition:

- multiplication:

 with the addition and multiplication is the field of complex numbers. From another of view,
with the addition and multiplication is the field of complex numbers. From another of view,  with complex additions and multiplication by real numbers is a 2-dimesional vector space.
with complex additions and multiplication by real numbers is a 2-dimesional vector space.
To perform basic computations it is convenient to introduce the imaginary unit, i=(0,1).[1] It has the property  Any complex number
Any complex number  can be written as
can be written as  (this is often called the algebraic form) and vice-versa. The numbers a and b are called the real part and the imaginary part of z, respectively. We denote
(this is often called the algebraic form) and vice-versa. The numbers a and b are called the real part and the imaginary part of z, respectively. We denote  and
and  Notice that i makes the multiplication quite natural:
Notice that i makes the multiplication quite natural:

The square root of number in the denominator in the above formula is called the modulus of z and denoted by  ,
,

We have for any two complex numbers  and
and 

 provided
provided 

For  we define also
we define also  , the conjugate, by
, the conjugate, by  Then we have
Then we have


 provided
provided 

- Geometric interpretation
Complex numbers may be naturally represented on the complex plane, where  corresponds to the point (x,y), see the fig. 1.
corresponds to the point (x,y), see the fig. 1.
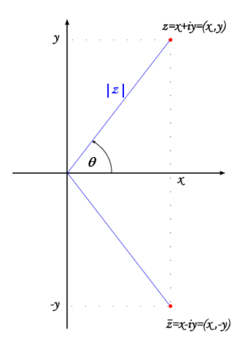
Fig. 1. Graphical representation of a complex number and its conjugate
Obviously, the conjugation is just the symmetry with respect to the x-axis.
- Trigonometric form
As the graphical representation suggests, any complex number z=a+bi of modulus 1 (i.e. a point from the unit circle) can be written as  for some
for some  So actually any (non-null)
So actually any (non-null)  can be represented as
can be represented as
 where r traditionally stands for |z|.
where r traditionally stands for |z|.
This is the trigonometric form of the complex number z. If we adopt convention that  then such
then such  is unique and called the argument of z.[2]
Graphically, the number
is unique and called the argument of z.[2]
Graphically, the number  is the (oriented) angle between the x-axis and the interval containing 0 and z.
is the (oriented) angle between the x-axis and the interval containing 0 and z.
- ↑ in some applications it is denoted by j as well.
- ↑ In literature the convention
![{\displaystyle \theta \in (-\pi ,\pi ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2742d923047f035ec3e8db8259485fda0629104b) is found as well.
is found as well.

































![{\displaystyle \theta \in (-\pi ,\pi ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2742d923047f035ec3e8db8259485fda0629104b)