Euclid's Elements: Difference between revisions
imported>Paul Wormer No edit summary |
imported>Paul Wormer |
||
| Line 3: | Line 3: | ||
The ''Elements'' is often considered as one of the documents, next to the Bible, that had the most impact on the Western culture. However, according to modern mathematical standards of rigor, the ''Elements'' show some shortcomings. These have been repaired as late as the 1890s by the German mathematician [[David Hilbert|Hilbert]]. | The ''Elements'' is often considered as one of the documents, next to the Bible, that had the most impact on the Western culture. However, according to modern mathematical standards of rigor, the ''Elements'' show some shortcomings. These have been repaired as late as the 1890s by the German mathematician [[David Hilbert|Hilbert]]. | ||
==Contents== | ==Contents== | ||
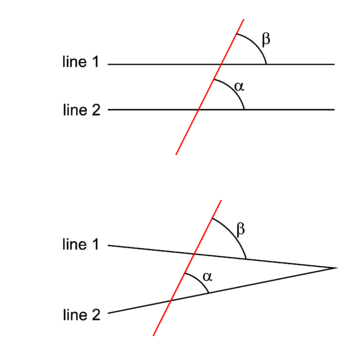
[[Image:Euclids parallel axiom.png|right|thumb|350px|Euclid's parallel axiom]] | [[Image:Euclids parallel axiom.png|right|thumb|350px|Euclid's parallel axiom. In the upper figure the angles α and β are equal. In the lower figure β > α.]] | ||
The work consists of thirteen ''Books''. The books I-V are devoted to planar geometry and the books X1-XIII to stereometry. The other books deal with the geometric foundation of the principles of numbers. | The work consists of thirteen ''Books''. The books I-V are devoted to planar geometry and the books X1-XIII to stereometry. The other books deal with the geometric foundation of the principles of numbers. | ||
The first 28 theorems are based on four evident assumptions, the [[axioms]], and treat mainly congruence of triangles and of other geometric figures. One of the most famous of theorems is the sixteenth which states that the exterior angle of a triangle is larger than any of the opposite interior angles. See the lower part of the figure, where angle β is larger than angle α by virtue of Euclid's sixteenth theorem. | The first 28 theorems are based on four evident assumptions, the [[axioms]], and treat mainly congruence of triangles and of other geometric figures. One of the most famous of theorems is the sixteenth which states that the exterior angle of a triangle is larger than any of the opposite interior angles. See the lower part of the figure, where angle β is larger than angle α by virtue of Euclid's sixteenth theorem. | ||
This theorem implies the existence of parallel lines, see the upper part of the figure. The converse of the theorem reads: if a straight line (the red one) meeting two other straight lines makes two alternate angles that are equal (α = β), then the two straight lines must be parallel. Euclid realized that this converse theorem could not be proven on basis of his four axioms, and therefore he added his fifth most famous axiom: | This theorem implies the existence of parallel lines, see the upper part of the figure. The converse of the theorem reads: if a straight line (the red one) meeting two other straight lines makes two alternate angles that are equal (α = β), then the two straight lines must be parallel. Euclid realized that this converse theorem could not be proven on basis of his four axioms, and therefore he added his fifth most famous axiom: if α ≠ β then line 1 and line 2 cross somewhere, that is, they are not parallel. | ||
'''(to be continued)''' | '''(to be continued)''' | ||
Revision as of 06:13, 24 January 2008
Euclid's Elements is the oldest systematic treatise on geometry. For more than twenty centuries the Elements was the major textbook model in the study and teaching of mathematics. Also in other fields Euclid's work led the way. The philosopher Spinoza wrote his work Ethics along the lines of the Elements and so did the physicist Newton when he composed his opus magnum Principia.
The Elements is often considered as one of the documents, next to the Bible, that had the most impact on the Western culture. However, according to modern mathematical standards of rigor, the Elements show some shortcomings. These have been repaired as late as the 1890s by the German mathematician Hilbert.
Contents
The work consists of thirteen Books. The books I-V are devoted to planar geometry and the books X1-XIII to stereometry. The other books deal with the geometric foundation of the principles of numbers.
The first 28 theorems are based on four evident assumptions, the axioms, and treat mainly congruence of triangles and of other geometric figures. One of the most famous of theorems is the sixteenth which states that the exterior angle of a triangle is larger than any of the opposite interior angles. See the lower part of the figure, where angle β is larger than angle α by virtue of Euclid's sixteenth theorem.
This theorem implies the existence of parallel lines, see the upper part of the figure. The converse of the theorem reads: if a straight line (the red one) meeting two other straight lines makes two alternate angles that are equal (α = β), then the two straight lines must be parallel. Euclid realized that this converse theorem could not be proven on basis of his four axioms, and therefore he added his fifth most famous axiom: if α ≠ β then line 1 and line 2 cross somewhere, that is, they are not parallel.
(to be continued)