Gamma function

The gamma function is a mathematical function that extends the domain of factorials to non-integers. The factorial of a positive integer n, written n!, is the product 1·2·3···n. The gamma function, denoted by $\Gamma$, is defined to satisfy $\Gamma (n)=(n-1)!$ for all positive integers n and to smoothly interpolate the factorial between the integers. The gamma function is one of the most commonly occurring examples of a nonelementary function; that is, a function that cannot be expressed in finite terms using algebraic operations, exponentials, and logarithms. Its study dates back to Leonhard Euler, who gave a formula for its calculation in 1729.

Motivation

It is easy to graphically interpolate the factorial to non-integer values, but is there a formula that describes the resulting curve?

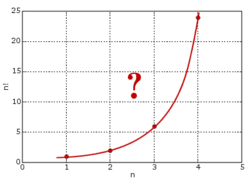
Is there a "closed-form expression" for the product 1·2·3···n? To be in closed form, the number of arithmetic operations should not depend on the size of n. Such an expression would have several uses; first of all, we could calculate n! in a less tedious manner than by performing (n-1) multiplications. As a famous anecdote tells, Carl Friedrich Gauss found as a child that the sum $1+2+...+n$ can be written as $n(n+1)/2$ — and was thereby able to quickly sum all the integers between 1 and 100, to the astonishment of his teacher. But a closed-form expression has more benefits than just computational convenience. If a formula does not depend on the size of n, it is also irrelevant whether the n is precisely an integer, and the formula should work for fractional numbers. Gauss's formula for sums of consecutive integers (such sums are now called triangular numbers) is obviously valid for non-integer values of n — in fact, when plotted for a continuously changing variable n, it describes a parabola.

Does it at all make sense to define the factorial of a fraction? Whether or not such numbers are useful, it is easy to see that they could at least be defined. By plotting the factorial of a few small integers, it becomes apparent that the dots can be connected with a smooth curve. We could, very roughly, calculate fractional factorials by just inspecting the graph. The problem is that it is not so easy to find a formula that exactly describes the curve. In fact, we now know that no simple such formula exists — "simple" meaning that no finite combination of elementary functions such as the usual arithmetic operations and the exponential function will do. Considering the simple solution for triangular numbers, it may come as surprise that changing "+" to "·" makes such a difference. But it is possible to find a general formula for factorials, if we deploy tools from calculus. As it turns out, the idea of a fractional factorial makes a lot of sense.

Defining the gamma function

The problem of extending the factorial to non-integer arguments was apparently first considered by Daniel Bernoulli and Christian Goldbach in the 1720s, and given its solution by the Swiss mathematician Leonhard Euler. The gamma function is commonly defined by a definite integral due to Euler,

$\Gamma (z)=\int _{0}^{\infty }e^{-t}t^{z-1}dt,$

where $t^{z-1}$ is interpreted as $e^{(z-1)\log t}$ if $z$ is not an integer. Using standard theorems from mathematical analysis, it can be shown that Euler's integral defines $\Gamma$ to be a continuous function if z is positive, or indeed any complex number with positive real part. To see that it corresponds to the factorial at integers, we can insert z+1 instead of z and perform an integration by parts to obtain

$\Gamma (z+1)=\left[-e^{-t}t^{z}\right]_{0}^{\infty }+z\int _{0}^{\infty }e^{-t}t^{z-1}dt=z\Gamma (z).$

This relation is called the recurrence formula or recurrence relation of the gamma function. The equation $f(z+1)=zf(z)$ is an example of a functional equation — an equation to be solved for the function f for all values of z. It is analogous to the recurrence satisfied by factorials, $n!=n(n-1)!$, the only difference being that the function argument has been shifted by 1. A repeated application of the gamma function's recurrence formula gives

$\Gamma (z+n)=z(z+1)(z+2)\cdots (z+n-1)\Gamma (z),$

which together with the initial value $\textstyle \Gamma (1)=\int _{0}^{\infty }e^{-t}dt=1$ establishes that

$\Gamma (n)=1\cdot 2\cdot 3\ldots (n-1)=(n-1)!$

for positive integers n. We can of course equivalently write $\Gamma (n+1)=n!$. We may use these formulas to explicitly calculate $\Gamma (n)$ or, conversely, to define z! for non-integers in terms of the gamma function. Euler's integral does not converge for $z\leq 0$, but by rewriting the recurrence relation as

$\Gamma (z)={\frac {\Gamma (z+n)}{z(z+1)\cdots (z+n-1)}},$

which we might call the forward recurrence relation, and choosing n such that z+n is positive, we can formally define $\Gamma (z)$ for negative values of z.

Uniqueness

This establishes that it is possible to define a continuous extension of the factorial, but an important question needs to be addressed: is the gamma function as we have defined it the unique such extension? The answer is no: there are infinitely many ways to connect any discrete set of points with a smooth curve. Infinitely many solutions remain if we demand that $\Gamma (z+1)=z\Gamma (z)$ must hold for all numbers z, not just integers, so this extra criterion is not strong enough.

The pragmatic reason for using Euler's particular extension of the factorial is that his integral often turns up in applications, whereas other extensions do not.

There is a complication, however: although Euler's function is arguably the most natural extension of the factorial, his integral is not the only formula that can be used to represent it. Other mathematicians who studied the gamma function have used different formulas as definitions, and although those formulas describe the same function, it is not entirely straightforward to prove the equivalence. Euler himself gave two different definitions: the first was not his integral but an infinite product,

$n!=\prod _{k=1}^{\infty }{\frac {\left(1+{\frac {1}{k}}\right)^{n}}{1+{\frac {n}{k}}}},$

of which he informed Goldbach in a letter dated October 13, 1729. He wrote to Goldbach again on January 8, 1730, to announce his discovery of the integral representation

$n!=\int _{0}^{1}(-\log s)^{n}\,ds,$

which is valid for n > 0. By the change of varibles $t=-\log s$, this becomes the familiar "Euler integral". A contemporary of Euler, James Stirling, also attempted to find a continuous expression for the factorial, using an entirely different approach. In his Methodus Differentialis (1730), he published the famous Stirling's formula

$n!\approx {\sqrt {2\pi n}}\left({\frac {n}{e}}\right)^{n}$

Although Stirling's formula gives a good estimate of n!, also for non-integers, it does not provide the exact value. Stirling made several attempts to refine his approximation, and eventually found a solution, although he never managed to prove that the extended version of his formula indeed corresponds exactly to the factorial. A proof was first given by Charles Hermite in 1900.

A definite characterization of the gamma function was not given until 1922. Harald Bohr and Johannes Mollerup then proved what is known as the Bohr-Mollerup theorem: that the gamma function is the unique solution to the factorial recurrence relation that is also logarithmically convex for positive z. That is, if a function interpolates the factorial, and its logarithm is a convex function for positive z, it must be the gamma function. The gamma function is also uniquely defined for negative numbers, and everywhere else in the complex plane, since it can be shown to be an analytic function and analytic functions are uniquely defined by analytic continuation from any region of the complex plane.

The Bohr-Mollerup theorem is useful because it is relatively easy to prove logarithmic convexity for any of the different formulas used to define the gamma function. Taking things further, instead of defining the gamma function by any particular formula, we can choose the conditions of the Bohr-Mollerup theorem as the definition, and then pick any formula we like that satisfies the conditions as a starting point for studying the gamma function. This approach was used by the Bourbaki group.

Notation

The name gamma function and the symbol $\Gamma$ were introduced by Adrien-Marie Legendre around 1811; Legendre also rewrote Euler's integral definition in its modern form. Although the symbol is an upper-case Greek Gamma, there is no accepted standard for whether the function name should be written "Gamma function" or "gamma function" (some authors simply write "$\Gamma$-function"). The alternative "Pi function" notation $\Pi (z)=z!$ due to Gauss is sometimes encountered in older literature, but Legendre's notation is dominant in modern works.

It is justified to ask why we distinguish between the "ordinary factorial" and the gamma function by using distinct symbols, and particularly why the gamma function should be normalized to $\Gamma (n+1)=n!$ instead of simply using "$\Gamma (n)=n!$". Legendre's motivation for the normalization does not appear to be known, and has been criticized as cumbersome by some (the 20th-century mathematician Cornelius Lanczos, for example, called it "void of any rationality" and would instead use $z!$). The normalization does simplify some formulas, but complicates others.

Main properties

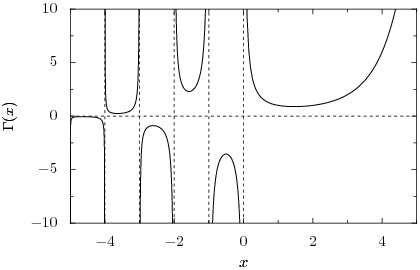
The behavior of $\Gamma (z)$ for an increasing positive variable is simple: it grows quickly — faster than an exponential function, asymptotically approaching the function given by Stirling's formula as $z\to \infty$.

The behavior as $z\to -\infty$ is more intricate. Euler's integral is the most common form encountered for the gamma function in applications, but to study the function's basic properties — such as its behavior for negative numbers, a product representation may be more convenient. We can use the product originally given by Euler, or either of the formulas

$\Gamma (z)=\lim _{m\to \infty }{\frac {m^{z}m!}{z(z+1)(z+2)\cdots (z+m)}}$

and

$\Gamma (z)={\frac {e^{-\gamma z}}{z}}\prod _{k=1}^{\infty }\left(1+{\frac {z}{k}}\right)^{-1}e^{z/k},\,\!$

where $\gamma \approx 0.577216$ is Euler's constant. Both representations can be derived from Euler's product representation by algebraic manipulation; the first was used by Gauss in 1811 in his study of the gamma function and the second by Karl Weierstrass. We could also consider what we called the forward recurrence relation to be a product representation. In any case, all these formulas directly reveal the behavior of the gamma function for negative numbers. Each product contains a factor that blows up — due to division by zero — as z approaches any of the integers $0,-1,-2,\ldots$. Thus, the gamma function must be undefined at those points; it is a meromorphic function with poles at the nonpositive integers. The following image shows the graph of the gamma function along the real line:

The gamma function is nonzero everywhere along the real line, although it comes arbitrarily close as $z\to -\infty$. There is in fact no complex number z for which $\Gamma (z)=0$, and hence the reciprocal gamma function $1/\Gamma$ is an entire function, with zeros at $z=0,-1,-2,\ldots$. We see that the gamma function has a local minimum at $x_{\mathrm {min} }\approx 1.46163$ where it attains the value $\Gamma (x_{\mathrm {min} })\approx 0.885603$. The gamma function must alternate sign between the poles because the product in the forward recurrence contains an odd number of negative factors if the number of poles between z and z+n is odd, and an even number if the number of poles is even.

Weierstrass originally wrote his product as one for $1/\Gamma$, in which case it is taken over the function's zeros rather than its poles. Inspired by this result, he proved what is known as the Weierstrass factorization theorem — that any entire function can be written as a product over its zeros; a generalization of the fundamental theorem of algebra.

The gamma function on the real line

Interval	Sign	Local extreme xm	Γ(xm)
(0, ∞)	+	1.4616321449683623413	0.88560319441088870028
(-1, 0)	-	-0.50408300826445540926	-3.5446436111550050891
(-2, -1)	+	-1.5734984731623904588	2.3024072583396801358
(-3, -2)	-	-2.6107208684441446500	-0.88813635840124192010
(-4, -3)	+	-3.6352933664369010979	0.24512753983436625044

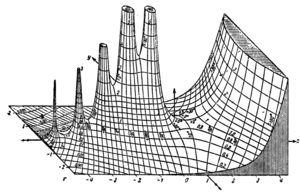
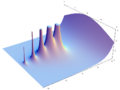
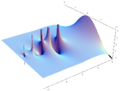
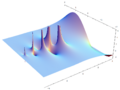
Although Euler was a pioneer in the theory of complex variables, he does not appear to have considered the factorial of a complex numbers, as instead Gauss first did. Plotting the gamma function in the complex plane yields beautiful graphs:

• 

  Absolute value

• 

  Real part

• 

  Imaginary part

An important property of the gamma function is the reflection formula

$\Gamma (z)\Gamma (1-z)={\frac {\pi }{\sin \pi z}}$

which gives a concise relation between the gamma function of positive and negative numbers. The division by a sine, which is periodically zero, again indicates the existence of the gamma function's periodically occurring poles. Further, inserting z = 1/2 reveals the surprising fact that

$\Gamma (1/2)={\sqrt {\pi }}.$

Hence, by the recurrence formula, the gamma function or factorial of any half-integer is a rational multiple of ${\sqrt {\pi }}$. Both results were discovered by Euler, and are most easily derived by comparing his product representation with his product formula for the sine function. The value of $\Gamma (1/2)$ can also be calculated from Euler's integral, by changing variables to obtain a Gaussian integral.

Multiplicative structure

Part of the mathematical significance of the gamma function comes from the multiplicative nature of its functional equations: the recurrence formula relates values of the gamma function separated by an integer distance by means of a product, while the reflection formula describes the product of the symmetric $\Gamma (z)$ and $\Gamma (-z)$. Gauss proved a new functional equation of the gamma function, the multiplication theorem

$\Gamma (z)\;\Gamma \left(z+{\frac {1}{k}}\right)\;\Gamma \left(z+{\frac {2}{k}}\right)\cdots \Gamma \left(z+{\frac {k-1}{k}}\right)=(2\pi )^{(k-1)/2}\;k^{1/2-kz}\;\Gamma (kz).\,\!$

of which the duplication formula

$\Gamma (z)\;\Gamma \left(z+{\frac {1}{2}}\right)=2^{1-2z}\;{\sqrt {\pi }}\;\Gamma (2z).\,\!$

is a special case. From the multiplication theorem, we can deduce identities involving multiple gamma function-values at rational numbers, such as

$2\pi ={\sqrt {3}}\;\Gamma \left({\begin{matrix}{\frac {1}{3}}\end{matrix}}\right)\Gamma \left({\begin{matrix}{\frac {2}{3}}\end{matrix}}\right)={\sqrt {2}}\;\Gamma \left({\begin{matrix}{\frac {1}{4}}\end{matrix}}\right)\Gamma \left({\begin{matrix}{\frac {3}{4}}\end{matrix}}\right).$

Given the curious appearance of $\pi$ in the formula for the gamma function of half an integer, it is worth asking whether any other individual numbers of the form $\Gamma (m/n)$ (where m and n are integers) have a simple formula. Numerically, we can calculate that

$\Gamma (1/3)=2.6789385347077476337$

$\Gamma (1/4)=3.6256099082219083119$

$\Gamma (1/5)=4.5908437119988030532$

...

but what are these numbers? Whatever they are, they are not known to be expressible in any simple way in terms of the elementary mathematical constants π and e. It has been proved that $\Gamma (n+r)$ is algebraically independent of π and itself a transcendental number for any integer n and each of the fractions r = 1/6, 1/4, 1/3, 2/3, 3/4, and 5/6. The case of $\Gamma (n+1/5)$ is unsettled, but at least two of the three numbers $\Gamma (1/5)$, $\Gamma (2/5)$ and $\pi$ must be algebraically independent. It is also known that $\Gamma (1/4)/\pi ^{1/4}$ is transcendental; that $\Gamma (1/4)$ is algebraically independent of $e^{\pi }$; that $\Gamma (1/3)$ is algebraically independent of $e^{\pi {\sqrt {3}}}$; and that neither $\Gamma (1/3)$ nor $\Gamma (1/4)$ is a Liouville number. There are many things that these numbers are not!

But there is more to be said. The values of the gamma function at rational numbers other than n/2 — at least, for values of the form n/24, do have geometrical significance similar to that of $\pi$: they correspond to special values of elliptic integrals, which are used to calculate the circumference of ellipses ($\pi$, of course, comes from the special case of an ellipse with equal axes). The number $\Gamma (1/4)$ also turns up if we try to calculate the arc length of a lemniscate, and is given by the arithmetic-geometric mean (agm) as

$\Gamma (1/4)={\sqrt {\frac {(2\pi )^{3/2}}{\mathrm {agm} (1,{\sqrt {2}})}}}.$

The connection between elliptic integrals, the gamma function and the arithmetic-geometric mean was first explored by Gauss. It has recently been used to derive extremely rapid algorithms for computing the numerical value of $\Gamma (n/24)$, of which the fastest known algorithm for computing $\pi$ can be viewed as a special case.

Derivatives and the zeta function

It is useful to think of the gamma function as the solution to its functional equations, particularly the recurrence relation. Most important special functions in applied mathematics arise as solutions to the continuous counterpart of recurrence relations: differential equations, which relate a function to its derivatives. The gamma function is useful in the study of many such functions — for instance, hypergeometric functions and Bessel functions, but does not by itself appear to satisfy any simple differential equation. Otto Hölder proved in 1887 that the gamma function at least does not satisfy any algebraic differential equation by showing that a solution to such an equation could not satisfy the gamma function's recurrence formula. This result is known as Hölder's theorem, and has been extended by later mathematicians.

Although the derivatives of the gamma function do not pop out of any simple differential equation, they exist and are analytic functions since the gamma function is analytic. They also turn out to have important applications. Taking the logarithm of Weierstrass's product, we can write the logarithm of the gamma function in the form of a series

$\log \Gamma (z)=-\log z-\gamma z+\sum _{k=1}^{\infty }\left[{\frac {z}{k}}-\log \left(1+{\frac {z}{k}}\right)\right].$

Differentiating termwise renders the logarithmic derivative of the gamma function

$\left(\log \Gamma (z)\right)'=-{\frac {1}{z}}-\gamma +\sum _{k=1}^{\infty }{\frac {1}{k}}-{\frac {1}{k+z}}$

which is called the digamma function, denoted by $\psi (z)\,\!$. The expression for the digamma function is simpler than the series we started with, all logarithms notably absent. If $z=n$, a positive integer, all but finitely many terms in the series cancel and we are left with

$\psi (n)=H_{n-1}-\gamma \,\!$

where $H_{n}$ is the harmonic number $\textstyle 1+{\frac {1}{2}}+{\frac {1}{3}}+\cdots +{\frac {1}{n}}$. As it generalizes harmonic numbers to non-integer indices, we could say that the digamma function is to harmonic numbers as the gamma function is to the factorials. Since $(\log f)'=f'/f$, we can recover the ordinary derivative of the gamma function as $\Gamma '(z)=\Gamma (z)\psi (z)$; the derivative at an integer is then $\Gamma '(n)=(H_{n-1}-\gamma )(n-1)!$ and in particular, $\Gamma '(1)=-\gamma$, providing a geometric interpretation of Euler's constant as the slope of the gamma function's graph at 1.

Continuing in a similar manner, it is possible to derive higher-order derivatives of the gamma function. We define the polygamma function (of order m) as

$\psi ^{(m-1)}(z)={\frac {d^{m}}{dz^{m}}}\log \Gamma (z).$

Of course, the digamma function is the special case $\psi =\psi ^{(0)}\,\;$.

The polygamma functions are related to the Riemann zeta function: it can be shown that the polygamma function at an integer value is expressible in terms of the zeta function at integers. With the higher-order derivatives available, it becomes possible to calculate the Taylor series of the gamma function around any integer in terms of Euler's constant and the zeta function. Choosing $1/\Gamma (z)$ instead of $\Gamma (z)$ for convenience, since the former is an entire function and hence has an everywhere convergent Taylor series in the simple point $z=0$, we can compute

${\frac {1}{\Gamma (z)}}=z+\gamma z^{2}+\left({\frac {\gamma ^{2}}{2}}-{\frac {\pi ^{2}}{12}}\right)z^{3}+\cdots =\sum _{k=1}^{\infty }a_{k}z^{k}$

where $a_{1}=1$, $a_{2}=\gamma$,

$a_{k}=ka_{1}a_{k}-a_{2}a_{k-1}+\sum _{j=2}^{k}(-1)^{j}\,\zeta (j)\,a_{k-j}\;\;\;\mathrm {for} \;k>2$

and $\zeta (s)\,\!$ is the Riemann zeta function.

This shows that the zeta function can be useful in the study of the gamma function, but a more important fact is that the gamma function can be used in the study of the zeta function. A fundamental property of the Riemann zeta function is its functional equation:

$\Gamma \left({\frac {s}{2}}\right)\zeta (s)\pi ^{-s/2}=\Gamma \left({\frac {1-s}{2}}\right)\zeta (1-s)\pi ^{(1-s)/2}.$

Among other things, this provides an explicit form for the analytic continuation of the zeta function to a meromorphic function in the complex plane and leads to an immediate proof that the zeta function has infinitely many so-called "trivial" zeros on the real line. Borwein et al. call this formula "one of the most beautiful findings in mathematics". Another champion for that title might be

$\zeta (z)\;\Gamma (z)=\int _{0}^{\infty }{\frac {t^{z-1}}{e^{t}-1}}\;dt.$

Both formulas were derived by Bernhard Riemann in his seminal 1859 paper "Über die Anzahl der Primzahlen unter einer gegebenen Grösse" ("On the Number of Prime Numbers less than a Given Quantity"), one of the milestones in the development of analytic number theory — the branch of mathematics that studies prime numbers using the tools of mathematical analysis. Factorial numbers, considered as discrete objects, are an important concept in classical number theory due to their compositeness properties, but Riemann found a use for their continuous extension that arguably turned out to be even more important.

Applications

Frequently encountered generalizations of the gamma function include the incomplete gamma functions

$\Gamma (a,x)=\int _{x}^{\infty }t^{a-1}\,e^{-t}\,dt,\,\!$

$\gamma (a,x)=\int _{0}^{x}t^{a-1}\,e^{-t}\,dt,\,\!$

and the beta function

$\mathrm {\mathrm {B} } (x,y)={\frac {\Gamma (x)\,\Gamma (y)}{\Gamma (x+y)}}.$

There are many other generalizations of the gamma function, including a multivariate gamma function and a q-series analog, the elliptic gamma function. Two analogs of the gamma function are the Barnes G-function, which extends superfactorials to the complex numbers, and the K-function which does the same for hyperfactorials. The Glaisher-Kinkelin constant sometimes appears in formulas related to these functions and the gamma function.

A variety of definite and indefinite integrals whose solutions are nonelementary can be expressed in terms of some combination of gamma functions and incomplete gamma functions. An example is that the Laplace transform of the power function x^c reduces to the Euler integral and hence the gamma function. Watson's triple integrals provide a more complicated example.

Integrands involving powers are particularly often susceptible to evaluation in terms of gamma functions, as it may be possible to consider the exponent an integer, eliminate it by repeated integration by parts to obtain a factorial times a solvable integral, and generalizing the result to arbitrary complex exponents by replacing the factorial with a gamma function. At other times, it is possible to directly transform an integral into an Euler integral by an appropriate change of variables.

Viewed as a non-elementary integral of an elementary function, the gamma function belongs to the same category of special functions as the error function, the exponential integral, the logarithmic integral, and the sine integral. There are many known interrelations between these functions and the gamma function.

The gamma function is also important in the theory of hypergeometric functions, the Meijer G-function and the Fox H-function. The G and H-functions are defined in terms of Mellin-Barnes integrals which are certain complex contour integrals of products of gamma functions.

The gamma function can be used to express many types of products besides the ordinary factorial, such as multiple factorials, Pochhammer symbols and binomial coefficients. For a monic polynomial P with roots p₁...p_n, one has the general formula

$\prod _{j=a}^{b}P(j)=\prod _{k=1}^{n}{\frac {\Gamma (b-p_{k}+1)}{\Gamma (a-p_{k})}},$

which in turn can be generalized to a formula for quotients of polynomials. Summation of series where each term is a rational function of the running index can be performed in an analogous manner using polygamma functions.

The gamma function is also used in statistics to define the gamma distribution, the inverse-gamma distribution, and the beta distribution.

Numerical calculation

It is a relatively convenient business to numerically calculate the gamma function for small arguments: given an approximation that holds on some interval of unit width, say for $1\leq z\leq 2$, or a complex strip with real part in such an interval, the value anywhere else can be computed easily via the recurrence and reflection formulas, using only a few multiplications or an evaluation of the sine in the reflection formula. In advanced numerical software that computes the gamma function with double precision (16 digits), the unit-interval approximation is usually an optimally chosen rational function, but for moderate precision an approximating polynomial works just as well. Abramowitz and Stegun give an approximating polynomial for the gamma function on $1\leq z\leq 2$ with an error less $10^{-6}$.

Alternatively, the gamma function can be computed directly from a Taylor series expansion such as that given in this article for $1/\Gamma$ (provided that one is able to compute the Riemann zeta function to obtain the coefficients), or even by direct numerical integration of Euler's integral. However, for fixed precision, either approach is generally less efficient than using a pre-computed approximation. None of the other series and product expansions given so far in this article converge quickly enough to be useful for practical computation.

For large arguments, say $|z|>10$, it is better to use an asymptotic expansion; the most popular is Stirling's series

$\Gamma (z+1)\sim {\sqrt {2\pi z}}\left({\frac {z}{e}}\right)^{z}\left(1+{\frac {1}{12z}}+{\frac {1}{288z^{2}}}-\cdots \right).$

This is Stirling's formula multiplied by a series that corrects the error. The series coefficients can be calculated in terms of Bernoulli numbers. The "$\sim$" sign denotes an asymptotic equality: the series diverges for every z, but yields arbitrarily accurate approximations of the gamma function as $z\to \infty$ if the series is truncated at the smallest term. We can use Stirling's series to calculate the gamma function for small numbers as well, using the recurrence relation. Taking $n=10$ and calculating $\Gamma (z)$ from $\Gamma (z+n)$, using the three first terms in Stirling's series, we obtain

$\Gamma (1)\approx 1.00000267$

$\Gamma (2)\approx 1.00000201$

This particular approximation is good to five decimal places on the interval $1\leq z\leq 2$. With larger n and more terms, Stirling's series can be used to calculate the gamma function with arbitrary precision. Other practical methods for arbitrary-precision calculation include the Lanczos approximation and Spouge's approximation, which are both similar in form to Stirling's series but have different convergence characteristics.

Finally, an efficient and relatively simple method for arbitrary-precision evaluation of the gamma function can be obtained by breaking Euler's integral into two pieces and integrating by parts to expand the lower one as a series. This gives

$\Gamma (z)=N^{z}e^{-N}\sum _{k=0}^{\infty }{\frac {N^{k}}{z(z+1)\dots (z+k)}}+\int _{N}^{\infty }e^{-t}t^{z-1}dt$

If $1\leq \mathrm {Re} (z)\leq 2$, the error introduced by omitting the right-hand integral and truncating the series at $k=6N$ is at most $2Ne^{-N}$. However, some care is needed to avoid loss of significance in the summation.

The computational complexity of evaluating the gamma function with arbitrary precision appears to be higher than that of the elementary functions. Using the aforementioned series obtained from Euler's integral, the gamma function of a complex number with a small real part can be calculated to n-digit precision with time complexity $O(n^{1/2}\log ^{2}nM(n))$ where $M(n)$ is the complexity of n-digit multiplication. If the argument is a small rational number, binary splitting brings the complexity down to $O(\log ^{2}nM(n))$. For comparison, elementary functions have complexity $O(\log nM(n))$. For $\Gamma (n/24)$, the arithmetic-geometric mean method mentioned earlier in the article also attains the complexity $O(\log nM(n))$.

It should be noted that it may be convenient to work with the logarithm of the gamma function instead of the gamma function itself, since the raw gamma function grows quickly and can cause overflow in computer arithmetic. It is common to encounter a quotient of two large gamma function values, which is most safely computed by subtracting two logarithms. Stirling's, Lanczos' and Spouge's approximations can all be calculated directly in logarithmic form.

A hand-drawn graph of the absolute value of the complex gamma function, from Tables of Higher Functions by Jahnke and Emde.

Double-precision floating-point implementations of the gamma function and its logarithm are available in most scientific computing software and special functions libraries, for example Matlab, GNU Octave, and the GNU Scientific Library. The gamma function was also added to the C mathematics library (math.h) as part of the C99 standard, but is not implemented by all C compilers. Arbitrary-precision implementations are available in most computer algebra systems, such as Mathematica and Maple. Pari/GP, MPFR and MPFUN contain free arbitrary-precision implementations.

Although the gamma function can be calculated virtually as easily as any mathematically simpler function with a modern computer — even with a programmable pocket calculator — this was of course not always the case. Until the mid-20th century, mathematicians relied on hand-made tables, notably a table computed by Gauss in 1813 and another by Legendre from 1825. Tables as well as hand-drawn graphs of the complex gamma function can be found in Tables of Higher Functions by Jahnke and Emde, first published in Germany in 1909.

There was in fact little practical need for anything but real values of the gamma function until the 1930s, when applications for the complex gamma function were discovered in theoretical physics. As electronic computers became available for the production of tables in the 1950s, several extensive tables for the complex gamma function were published to meet the demand, including a table accurate to 12 decimal places from by the U.S. National Bureau of Standards.

Concluding remarks

The theory of the gamma function and its applications is rich enough to fill books — indeed, such books have been written. A recommended reading, although the book is now out of print, is Emil Artin's 38-page exposition The Gamma Function from 1964. Artin, who argues that "the gamma function can be thought of as one of the elementary functions", develops the theory of the gamma function of a real variable in a thorough way using only elementary methods.

The gamma function has caught the interest of some of the most prominent mathematicians of all time. Its history reflects many of the major developments within mathematics since the 18th century. In the words of P. J. Davis, "each generation has found something of interest to say about the gamma function. Perhaps the next generation will also." The Nobel Prize-winning physicist Gerard 't Hooft urges prospective students of physics to "enjoy studying its properties".