Clausius-Clapeyron relation: Difference between revisions
imported>Paul Wormer (I and II roman) |
mNo edit summary |
||
| (20 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
{{subpages}} | {{subpages}} | ||
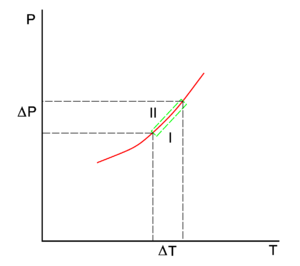
{{Image|Clapeyron.png|right|300px|The red line in the P-T diagram is the coexistence curve of two phases, I and II, of a single system. Phase | {{Image|Clapeyron.png|right|300px|The red line in the P-T diagram is the coexistence curve of two phases, I and II, of a single-component system. Phase I may be the vapor and II the liquid phase of the component. The lower green line gives the slope in phase I and the upper green line in phase II.}} | ||
The ''' | The '''Clausius-Clapeyron relation''' is an equation for a single-component system<ref>See J.C.M. Li, ''Clapeyron Equation for Multicomponent Systems'', Journal of Chemical Physics, vol. '''25''', pp. 572–574 (1956) for a generalization to systems of more than one component.</ref> | ||
consisting of two phases in thermodynamic equilibrium at constant absolute [[temperature]] ''T'' and constant [[pressure]] ''P''. A curve in a two-dimensional thermodynamical diagram that separates two phases in equilibrium is known as a [[coexistence curve]]. The Clausius–Clapeyron relation gives the [[slope]] of the coexistence curve in the ''P''-''T'' diagram: | |||
:<math> | :<math> | ||
\frac{\mathrm{d}P}{\mathrm{d}T} = \frac{Q}{T\,(V^{\mathrm{ | \frac{\mathrm{d}P}{\mathrm{d}T} = \frac{Q}{T\,(V^{\mathrm{I}} - V^{\mathrm{II}})} , | ||
</math> | </math> | ||
where ''Q'' is the molar heat of transition. It is the heat necessary to bring one mole of the compound from phase | where ''Q'' is the molar heat of transition. It is the heat necessary to bring one mole of the compound constituting the system from phase II into phase I; it is also known as ''latent heat''. For phase transitions with constant ''P'', it is the molar transition [[enthalpy]]. For instance, when phase II is a liquid and phase I is a vapor, then ''Q'' ≡ ''H''<sub>v</sub> is the molar [[heat of vaporization]] (also known as molar enthalpy of evaporation). Further, ''V''<sup> I</sup> is the [[molar volume]] of phase I at the pressure ''P'' and temperature ''T'' of the point where the slope is considered and ''V''<sup> II</sup> is the same for phase II. | ||
The equation is named after [[Émile Clapeyron]], who | The equation is named after [[Émile Clapeyron]], who published it in 1834, and [[Rudolf Clausius]], who put it on firm thermodynamic basis in 1850. | ||
==Derivation== | ==Derivation== | ||
| Line 44: | Line 45: | ||
-S^{\mathrm{I}} \Delta T +V^{\mathrm{I}} \Delta P = -S^{\mathrm{II}} \Delta T +V^{\mathrm{II}} \Delta P | -S^{\mathrm{I}} \Delta T +V^{\mathrm{I}} \Delta P = -S^{\mathrm{II}} \Delta T +V^{\mathrm{II}} \Delta P | ||
\;\Longrightarrow\; | \;\Longrightarrow\; | ||
\frac{\Delta P}{\Delta T} = \frac{S^{\mathrm{ | \frac{\Delta P}{\Delta T} = \frac{S^{\mathrm{I}} - S^{\mathrm{II}}}{V^{\mathrm{I}} - V^{\mathrm{II}}}. | ||
</math> | </math> | ||
From the second law of thermodynamics it is known that for a reversible phase transition it holds that | From the second law of thermodynamics it is known that for a reversible phase transition it holds that | ||
:<math> | :<math> | ||
S^{\mathrm{ | S^{\mathrm{I}} - S^{\mathrm{II}} = \frac{Q}{T}, | ||
</math> | </math> | ||
where ''Q'' is the amount of heat necessary to convert one mole of | where ''Q'' is the amount of heat necessary to convert one mole of | ||
compound from phase | compound from phase II into phase I. Elimination of the entropy and taking the limit of infinitesimally small changes in ''T'' and ''P'' gives the ''Clausius-Clapeyron | ||
equation'', | equation'', | ||
:<math> | :<math> | ||
\frac{dP}{dT} = \frac{Q}{T(V^{\mathrm{ | \frac{dP}{dT} = \frac{Q}{T(V^{\mathrm{I}} - V^{\mathrm{II}})} . | ||
</math> | </math> | ||
==Approximate solution== | ==Approximate solution== | ||
The Clausius-Clapeyron equation is exact. When | The Clausius-Clapeyron equation is exact. When the following assumptions are made, it may be integrated: | ||
* The [[molar volume]] of phase | * The [[molar volume]] of phase II is negligible compared to the molar volume of phase I: ''V''<sup> I</sup> >> ''V''<sup> II</sup>. In general, far from the [[critical point]], this inequality holds well for liquid-gas equilibriums. | ||
* Phase | * Phase I satisfies the [[ideal gas law]], where ''R'' is the [[molar gas constant]], | ||
::<math> | ::<math> | ||
PV^{\mathrm{ | PV^{\mathrm{I}} = R T. \, | ||
</math> | </math> | ||
:If phase | :If phase I is a gas and the pressure is fairly low, this assumption is reasonable. | ||
* The transition (latent) heat ''Q'' is constant over the temperature integration interval. The integrations run from the lower temperature ''T''<sub>1</sub> to the upper temperature ''T''<sub>2</sub> and from ''P''<sub>1</sub> to ''P''<sub>2</sub>. | * The transition (latent) heat ''Q'' is constant over the temperature integration interval. The integrations run from the lower temperature ''T''<sub>1</sub> to the upper temperature ''T''<sub>2</sub> and from ''P''<sub>1</sub> to ''P''<sub>2</sub>. | ||
| Line 80: | Line 82: | ||
\frac{dT}{T^2} | \frac{dT}{T^2} | ||
\;\Longrightarrow\; | \;\Longrightarrow\; | ||
\ln\left(\frac{P_2}{P_1}\right) = | \ln\left(\frac{P_2}{P_1}\right) = \frac{Q}{R}\;\left( \frac{1}{T_1} - \frac{1}{T_2} \right), | ||
</math> | |||
where ln(''P''<sub>2</sub>/''P''<sub>1</sub>) is the natural (base ''e'') [[logarithm]] of ''P''<sub>2</sub>/''P''<sub>1</sub>. We reiterate that for a gas-liquid equilibrium ''Q'' = ''H''<sub>''v''</sub>, the [[heat of vaporization]]. | |||
For example, at the top of Mount Everest, atmospheric pressure is about a third of its value at sea level. Using the values R = 8.3145 J/K and Q = 40.65 kJ/mol, the equation gives T<sub>2</sub> = 1/[1/373.16 + 8.3145 ln(3) / (40.65 10<sup>3</sup>) ] = 344 K (71 °C) for the boiling temperature of water. It takes many hours to boil an egg on top of the Mount Everest in water of 71 °C. | |||
==Application== | |||
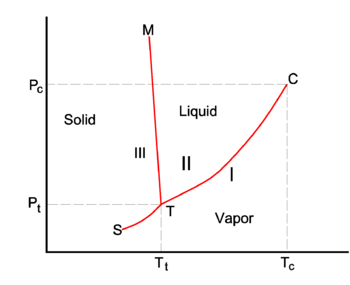
{{Image|Phase diagram.png|right|350px|''P''-''T'' diagram of water. Schematic and qualitative.}} | |||
In the figure on the right an impression of part of the phase diagram of [[water]] (H<sub>2</sub>O) is shown. The three phases are: I (vapor), II (liquid) and III (solid). The Clausius-Clapeyron equation | |||
:<math> | |||
\frac{dP}{dT} = \frac{Q}{T(V^\mathrm{I}-V^\mathrm{II})} | |||
</math> | </math> | ||
gives the slope of the three coexistence (equilibrium, red) curves. The boiling line T-C has a positive slope, because the heat of vaporization ''Q'' = ''H''<sub>v</sub> is positive and the molar volume of the vapor ''V''<sup> I</sup> is (much) larger than that of the liquid ''V''<sup> II</sup>. For a similar reason the sublimation (transition from solid to vapor) line T-S has a positive slope. The sublimation line T-S is steeper than the boiling line T-C because the heat of sublimation is larger than the heat of vaporization, while in the neighborhood of crossing point T (the [[triple point]]) the difference in molar volumes is nearly equal. The melting line T-M has a negative slope, which occurs only for a few compounds (among them water). Consider | |||
:<math> | |||
\frac{dP}{dT} = \frac{Q_\mathrm{melt}}{T(V^\mathrm{II}-V^\mathrm{III})} = \frac{S^\mathrm{II}- S^\mathrm{III}}{V^\mathrm{II}-V^\mathrm{III}} | |||
</math> | |||
The molar volume ''V''<sup>III</sup> of the solid is larger than the molar volume ''V''<sup>II</sup> of the liquid, while the enthalpy of melting ''Q''<sub>melt</sub> = ''S''<sup>II</sup>−''S''<sup>III</sup> > 0 (a liquid has a larger entropy than the corresponding solid, it costs energy to melt a solid). Compounds that have melting lines with negative slope, have a solid phase of lower density than the liquid phase. For example, it is very well-known that solid water (ice) floats on liquid water. | |||
The point C is the [[critical point]]. Beyond this point the vapor and the liquid are one indistinguishable fluid. There is no phase transition or a phase interface to the right of the melting line and above the line of constant ''P'' = ''P''<sub>c</sub>. The gas/liquid fluid also appears to the right of the line of constant ''T'' = ''T''<sub>c</sub>. | |||
For water the critical point C is at (''T''<sub>c</sub>, ''P''<sub>c</sub>) = (647.1 [[K]], 220.6 [[Bar (unit)|bar]]) (a bar is almost equal to an [[atmosphere (unit)|atmosphere]] and is 100 k[[Pascal (unit)|pascal]]) and the triple point T is at (''T''<sub>t</sub>, ''P''<sub>t</sub>) = (273.16 K, 6.1173 mbar). | |||
The Clausius-Clapeyron relation also holds for transitions between different crystalline phases. | |||
==Reference== | |||
<references />[[Category:Suggestion Bot Tag]] | |||
Latest revision as of 11:01, 29 July 2024
The Clausius-Clapeyron relation is an equation for a single-component system[1] consisting of two phases in thermodynamic equilibrium at constant absolute temperature T and constant pressure P. A curve in a two-dimensional thermodynamical diagram that separates two phases in equilibrium is known as a coexistence curve. The Clausius–Clapeyron relation gives the slope of the coexistence curve in the P-T diagram:
where Q is the molar heat of transition. It is the heat necessary to bring one mole of the compound constituting the system from phase II into phase I; it is also known as latent heat. For phase transitions with constant P, it is the molar transition enthalpy. For instance, when phase II is a liquid and phase I is a vapor, then Q ≡ Hv is the molar heat of vaporization (also known as molar enthalpy of evaporation). Further, V I is the molar volume of phase I at the pressure P and temperature T of the point where the slope is considered and V II is the same for phase II.
The equation is named after Émile Clapeyron, who published it in 1834, and Rudolf Clausius, who put it on firm thermodynamic basis in 1850.
Derivation
The condition of thermodynamical equilibrium at constant pressure P and constant temperature T between two phases I and II is the equality of the molar Gibbs free energies G,
The molar Gibbs free energy of phase α (α = I, II) is equal to the chemical potential μα of this phase. Hence the equilibrium condition can be written as,
which holds everywhere along the coexistence (red) curve in the figure.
If we go reversibly along the lower and upper green line in the figure [the tangents to the curve in the point (T,P)], the chemical potentials of the phases, being functions of T and P, change by ΔμI and ΔμII, for phase I and II, respectively, while the system stays in equilibrium,
From classical thermodynamics it is known that
Here Sα is the molar entropy (entropy per mole) of phase α and Vα is the molar volume (volume of one mole) of this phase. It follows that
From the second law of thermodynamics it is known that for a reversible phase transition it holds that
where Q is the amount of heat necessary to convert one mole of compound from phase II into phase I. Elimination of the entropy and taking the limit of infinitesimally small changes in T and P gives the Clausius-Clapeyron equation,
Approximate solution
The Clausius-Clapeyron equation is exact. When the following assumptions are made, it may be integrated:
- The molar volume of phase II is negligible compared to the molar volume of phase I: V I >> V II. In general, far from the critical point, this inequality holds well for liquid-gas equilibriums.
- Phase I satisfies the ideal gas law, where R is the molar gas constant,
- If phase I is a gas and the pressure is fairly low, this assumption is reasonable.
- The transition (latent) heat Q is constant over the temperature integration interval. The integrations run from the lower temperature T1 to the upper temperature T2 and from P1 to P2.
Under these condition the Clausius-Clapeyron equation becomes
Integration gives
where ln(P2/P1) is the natural (base e) logarithm of P2/P1. We reiterate that for a gas-liquid equilibrium Q = Hv, the heat of vaporization.
For example, at the top of Mount Everest, atmospheric pressure is about a third of its value at sea level. Using the values R = 8.3145 J/K and Q = 40.65 kJ/mol, the equation gives T2 = 1/[1/373.16 + 8.3145 ln(3) / (40.65 103) ] = 344 K (71 °C) for the boiling temperature of water. It takes many hours to boil an egg on top of the Mount Everest in water of 71 °C.
Application
In the figure on the right an impression of part of the phase diagram of water (H2O) is shown. The three phases are: I (vapor), II (liquid) and III (solid). The Clausius-Clapeyron equation
gives the slope of the three coexistence (equilibrium, red) curves. The boiling line T-C has a positive slope, because the heat of vaporization Q = Hv is positive and the molar volume of the vapor V I is (much) larger than that of the liquid V II. For a similar reason the sublimation (transition from solid to vapor) line T-S has a positive slope. The sublimation line T-S is steeper than the boiling line T-C because the heat of sublimation is larger than the heat of vaporization, while in the neighborhood of crossing point T (the triple point) the difference in molar volumes is nearly equal. The melting line T-M has a negative slope, which occurs only for a few compounds (among them water). Consider
The molar volume VIII of the solid is larger than the molar volume VII of the liquid, while the enthalpy of melting Qmelt = SII−SIII > 0 (a liquid has a larger entropy than the corresponding solid, it costs energy to melt a solid). Compounds that have melting lines with negative slope, have a solid phase of lower density than the liquid phase. For example, it is very well-known that solid water (ice) floats on liquid water.
The point C is the critical point. Beyond this point the vapor and the liquid are one indistinguishable fluid. There is no phase transition or a phase interface to the right of the melting line and above the line of constant P = Pc. The gas/liquid fluid also appears to the right of the line of constant T = Tc.
For water the critical point C is at (Tc, Pc) = (647.1 K, 220.6 bar) (a bar is almost equal to an atmosphere and is 100 kpascal) and the triple point T is at (Tt, Pt) = (273.16 K, 6.1173 mbar).
The Clausius-Clapeyron relation also holds for transitions between different crystalline phases.
Reference
- ↑ See J.C.M. Li, Clapeyron Equation for Multicomponent Systems, Journal of Chemical Physics, vol. 25, pp. 572–574 (1956) for a generalization to systems of more than one component.