Van der Waals equation: Difference between revisions
imported>Larry Sanger m (Unprotected "Van der Waals equation/Draft": Draft should be editable) |
mNo edit summary |
||
| (20 intermediate revisions by 8 users not shown) | |||
| Line 1: | Line 1: | ||
{{subpages}} | {{subpages}} | ||
In [[physics]], [[chemistry]], and [[chemical engineering]], the '''van der Waals equation''' is an | In [[physics]], [[chemistry]], and [[chemical engineering]], the '''van der Waals equation''' is an equation of state for a fluid composed of particles that have a non-zero size and a pairwise attractive interparticle [[force]] (such as the [[van der Waals force]]). It was derived by [[Johannes Diderik van der Waals]] in his doctoral thesis (Leiden 1873)<ref>van der Waals, JD (1873) ''Over de Continuiteit van den Gas- en Vloeistoftoestand (on the continuity of the gas and liquid state)''. Ph.D. thesis, Leiden, The Netherlands. [http://www.scs.uiuc.edu/~mainzv/exhibit/vanderwaals.htm]</ref> by modification of the [[ideal gas law]]. The importance of this work is the recognition that the gas and liquid phase of a substance transform continuously into each other. Above the [[critical temperature]], there is no difference between the gas and liquid phase, which is why it is proper to speak of the equation of a ''fluid'', a generic term for liquid ''and'' gas. | ||
==Equation== | ==Equation== | ||
| Line 12: | Line 12: | ||
:''R'' is the [[gas constant]], ''R'' = ''N''<sub>A</sub> ''k'', where ''k'' is the [[Boltzmann constant]] and ''N''<sub>A</sub> is [[Avogadro's constant]]. | :''R'' is the [[gas constant]], ''R'' = ''N''<sub>A</sub> ''k'', where ''k'' is the [[Boltzmann constant]] and ''N''<sub>A</sub> is [[Avogadro's constant]]. | ||
The van der Waals parameter ''b'' is proportional to ''N''<sub>A</sub> times the volume of a single particle—the volume bounded by the [[atomic radius]]. In van der Waals' original derivation, given below, ''b''/''N''<sub>A</sub> is | The van der Waals parameter ''b'' is proportional to ''N''<sub>A</sub> times the volume of a single particle—the volume bounded by the [[atomic radius]]. In van der Waals' original derivation, given below, ''b''/''N''<sub>A</sub> is 4 times the volume of a single particle. Particles are seen as hard spheres. Observe that the pressure ''p'' goes to infinity when the container is completely filled, so that there is no void space left for the particles to move. This occurs when ''V = n b''. | ||
Although the parameters ''a'' and ''b'' are referred to as "the Van der Waals constants", they are not truly constants because they vary from one gas to another; they are, however, independent of ''P'', ''V'', and ''T''. In other words, they are constant for the specific gas being considered. | |||
===Illustration=== | ===Illustration=== | ||
From the empirical | From the empirical critical temperature ''T''<sub>''C''</sub> and the empirical [[critical pressure]] ''p''<sub>''C''</sub> the constants ''a'' and ''b'' can be obtained by the equations | ||
:<math> | :<math> | ||
a = \frac{27}{64}\frac{(RT_C)^2}{p_C}\quad \hbox{and}\quad b = \frac{RT_C}{8p_C} | a = \frac{27}{64}\frac{(RT_C)^2}{p_C}\quad \hbox{and}\quad b = \frac{RT_C}{8p_C} | ||
</math> | </math> | ||
The [[ | The [[carbon dioxide]] molecule (CO<sub>2</sub>) has<ref>J. V. Sengers and J. M. H. Levelt-Sengers, | ||
Annual Review of Physical Chemistry, vol. '''37''', p. 189 (1986)</ref> ''p''<sub>''C''</sub> = 7.37 MPa and ''T''<sub>''C''</sub> = 304.1 K = 31 ˚C , which gives | Annual Review of Physical Chemistry, vol. '''37''', p. 189 (1986)</ref> ''p''<sub>''C''</sub> = 7.37 MPa and ''T''<sub>''C''</sub> = 304.1 K = 31 ˚C , which gives | ||
''a'' = 0.366 m<sup>6</sup> Pa and ''b'' = 42.9 10<sup>-6</sup> m<sup>3</sup>. For four different temperatures the van der Waals curves are given in the following figure. The critical pressure is at 73.7 bar = 7370 kPa (is close to 73.7 atm). | ''a'' = 0.366 m<sup>6</sup> Pa and ''b'' = 42.9 10<sup>-6</sup> m<sup>3</sup>. For four different temperatures the van der Waals curves are given in the following figure. The critical pressure is at 73.7 [[Bar (unit)|bar]] = 7370 kPa (is close to 73.7 [[Atmosphere (unit)|atm]]). | ||
{{Image|Vanderwaals_equation_of_state.png|center|500px|Different isotherms (curves of constant temperature) obeying the van der Waals equation of state. The dashed line from A to B is drawn in accordance with Maxwell's equal area rule.}} | |||
For large | For large volumes (on the right-hand side of the figure), the pressures are low and the fluid obeys approximately the [[ideal gas law]] ''pV'' = ''nRT'' for all temperatures shown. If we decrease the volume (go to the left in the figure along an isotherm), the pressure rises. Consider the (blue) [[isotherm]] of 10 <sup>0</sup>C, which is below the critical temperature. Decrease the volume until we reach the point ''B'', where condensation (formation of liquid CO<sub>2</sub>) starts. At this point the van der Waals curve is no longer physical (excluding the possibility of the occurrence of an oversaturated, metastable gas), because in reality from ''B'' to the left (to smaller volume), the pressure stays constant—equal to the [[vapor pressure]] of the liquid. The real physical behavior is given by the dashed line of gas-liquid coexistence. The areas, bounded by the 10 °C isotherm, below and above the coexistence line are equal. This is the content of <i>Maxwell's equal-area rule</i>. Decreasing the volume further, we end at point ''A'' where all molecules are now in the liquid phase, no molecules are remaining in the gas phase. When the volume is diminished of a vessel that contains only liquid, the pressure rises steeply, because the [[compressibility]] of a liquid is considerable smaller than that of a gas. | ||
If | If one follows the (green) 31 °C curve of critical temperature, one meets a horizontal point of inflection (first and second derivatives of ''p'' with respect to ''V'' are zero). Note that the figure exhibits two isotherms of temperature higher than the critical temperature; if they are followed, no liquid–gas phase transition will be seen; the higher pressure fluid will resemble a liquid, while at lower pressures the fluid will be more gaslike. At temperatures higher than the critical temperature, no gas–liquid interface occurs. | ||
Although the | Although the maxima and minima in the van der Waals curves below the critical point are not physical, the equation for these curves, derived by van der Waals in 1873, was a great scientific achievement. Even today it is not possible to give a ''single'' equation that describes correctly the gas–liquid phase transition. | ||
==Validity== | ==Validity== | ||
Above the | Above the critical temperature the van der Waals equation is an improvement of the ideal gas law, and for lower temperatures the equation is also qualitatively reasonable for the liquid state and the low-pressure gaseous state. However, the van der Waals model cannot be taken seriously in a quantitative sense; it is only useful for qualitative purposes.<ref>T. L. Hill, ''Statistical Thermodynamics'', Addison-Wesley, Reading (1960), p. 280</ref> | ||
In the [[phase transition|first-order phase transition]] range of ''(p,V,T)'' (where the [[liquid]] [[phase (matter)|phase]] and the [[gas]] phase are in equilibrium) it does not exhibit the empirical fact that ''p'' is constant (equal to the vapor pressure of the liquid) as a function of ''V'' for a given temperature. | In the [[phase transition|first-order phase transition]] range of ''(p,V,T)'' (where the [[liquid]] [[phase (matter)|phase]] and the [[gas]] phase are in equilibrium) it does not exhibit the empirical fact that ''p'' is constant (equal to the vapor pressure of the liquid) as a function of ''V'' for a given temperature. | ||
| Line 47: | Line 49: | ||
:<math> p = \frac{RT}{V_\mathrm{m}}.</math> | :<math> p = \frac{RT}{V_\mathrm{m}}.</math> | ||
{{Image|Van der Waals equation spheres.png|right|250px|The cyan colored spheres are two fluid particles, of diameter ''d'', in closest contact. The (black) enveloping sphere is excluded to other particles.}} | |||
Next assume that all particles are hard spheres of the same finite radius ''r'' = ''d''/2 (the [[van der Waals radius]]). The effect of the finite volume of the particles is to decrease the available void space in which the particles are free to move. We must replace ''V'' by ''V-b'', where ''b'' is called the ''excluded volume''. The corrected equation becomes | Next assume that all particles are hard spheres of the same finite radius ''r'' = ''d''/2 (the [[van der Waals radius]]). The effect of the finite volume of the particles is to decrease the available void space in which the particles are free to move. We must replace ''V'' by ''V-b'', where ''b'' is called the ''excluded volume''. The corrected equation becomes | ||
:<math> p = \frac{RT}{V_\mathrm{m}-b}.</math> | :<math> p = \frac{RT}{V_\mathrm{m}-b}.</math> | ||
The excluded volume ''b'' is not just equal to ''N''<sub>A</sub> times the volume occupied by a single particle, but actually 4 ''N''<sub>A</sub> times that volume. To see this we must realize that two colliding particles are enveloped by a sphere of radius ''d'' that is forbidden for the centers of the other particles, see the figure. If the center of a third particle would come into the enveloping sphere, it would mean that the third particle penetrates any of the other two, which, by definition, hard spheres are unable to do. The excluded volume ''b''/''N''<sub>A</sub> per particle pair is 4π/3 × ''d''<sup>3</sup> = 32π/3 × ''r''<sup>3</sup>, so that the excluded volume is '''not''' 8π/3 × ''r''<sup>3</sup> (the sum of volumes of the two particles), but ''four'' times as much. | The excluded volume ''b'' is not just equal to ''N''<sub>A</sub> times the volume occupied by a single particle, but actually 4 ''N''<sub>A</sub> times that volume. To see this we must realize that two colliding particles are enveloped by a sphere of radius ''d'' that is forbidden for the centers of the other particles, see the figure. If the center of a third particle would come into the enveloping sphere, it would mean that the third particle penetrates any of the other two, which, by definition, hard spheres are unable to do. The excluded volume ''b''/''N''<sub>A</sub> per particle pair is 4π/3 × ''d''<sup>3</sup> = 32π/3 × ''r''<sup>3</sup>, so that the excluded volume is '''not''' 8π/3 × ''r''<sup>3</sup> (the sum of volumes of the two particles), but ''four'' times as much.<ref>That the argument leading to this factor is not completely trivial appears from the fact that [[James Clerk Maxwell|Maxwell]] considered it to be erroneous; Maxwell derived a factor 16. See, A. Ya. Kipnis, B. E. Yavelov, and J. S. Rowlinson, ''Van der Waals and Molecular Science'', Oxford University Press, (1996) p. 64</ref> | ||
It was a point of concern to van der Waals that the factor | It was a point of concern to van der Waals that the factor 4 yields actually an upper bound; empirical values for ''b'' are usually lower. Of course molecules are not infinitely hard, as van der Waals assumed, but are often fairly soft. | ||
Next, we introduce a pairwise attractive force between the particles. Van der Waals assumed that, notwithstanding the existence of this force, the density of the fluid is homogeneous. | Next, we introduce a pairwise attractive force between the particles. Van der Waals assumed that, notwithstanding the existence of this force, the density of the fluid is homogeneous. Furthermore, he assumed that the range of the attractive force is so small that the great majority of the particles do not feel that the container is of finite size. That is, the bulk of the particles do not notice that they have more attracting particles to their right than to their left when they are relatively close to the left-hand wall of the container. The same statement holds with left and right interchanged. Given the homogeneity of the fluid, the bulk of the particles do not experience a net force pulling them to the right or to the left. This is different for the particles in surface layers directly adjacent to the walls. They feel a net force from the bulk particles pulling them into the container, because this force is not compensated by particles between them and the wall (another assumption here is that there is no interaction between walls and particles). This net force decreases the force exerted onto the wall by the particles in the surface layer. The net force on a surface particle, pulling it into the container, is proportional to the number density ''C'' ≡ ''N''<sub>A</sub>/''V''<sub>m</sub>. The number of particles in the surface layers is, again by assuming homogeneity, also proportional to the density. In total, the force on the walls is decreased by a factor proportional to the square of the density and the pressure (force per unit surface) is decreased by | ||
:<math>a'C^2= a' \left(\frac{N_\mathrm{A}}{V_\mathrm{m}}\right)^2 = \frac{a}{V_\mathrm{m}^2} | :<math>a'C^2= a' \left(\frac{N_\mathrm{A}}{V_\mathrm{m}}\right)^2 = \frac{a}{V_\mathrm{m}^2} | ||
\quad\hbox{with}\quad a \equiv a' N^2_\mathrm{A}, | \quad\hbox{with}\quad a \equiv a' N^2_\mathrm{A}, | ||
| Line 72: | Line 74: | ||
===Statistical thermodynamics derivation=== | ===Statistical thermodynamics derivation=== | ||
The canonical [[partition function]] ''Q'' of an ideal gas consisting of ''N = nN''<sub>A</sub> identical particles, is | The canonical [[partition function (statistical physics)|partition function]] ''Q'' of an ideal gas consisting of ''N = nN''<sub>A</sub> identical particles, is | ||
:<math> | :<math> | ||
Q = \frac{q^N}{N!}\quad \hbox{with}\quad q = \frac{V}{\Lambda^3} | Q = \frac{q^N}{N!}\quad \hbox{with}\quad q = \frac{V}{\Lambda^3} | ||
| Line 80: | Line 82: | ||
\Lambda = \sqrt{\frac{h^2}{2\pi m k T}} | \Lambda = \sqrt{\frac{h^2}{2\pi m k T}} | ||
</math> | </math> | ||
with the usual definitions: ''h'' is [[Planck's constant]], ''m'' the mass of a particle, ''k'' [[Boltzmann's constant]] and ''T'' the absolute temperature. In an ideal gas ''q'' is the partition function of a single particle in a vessel of volume ''V''. In order to derive the van der Waals equation we assume now that each particle moves independently in an average potential field offered by the other particles. The averaging over the particles is easy because we will assume that the particle density of the van der Waals fluid is homogeneous. | with the usual definitions: ''h'' is [[Planck's constant]], ''m'' the mass of a particle, ''k'' [[Boltzmann's constant]] and ''T'' the absolute temperature. In an ideal gas ''q'' is the partition function of a single particle in a vessel of volume ''V''. In order to derive the van der Waals equation, we assume now that each particle moves independently in an average potential field offered by the other particles. The averaging over the particles is easy because we will assume that the particle density of the van der Waals fluid is homogeneous. | ||
The interaction between a pair of particles, which are hard spheres, is taken to be | The interaction between a pair of particles, which are hard spheres, is taken to be | ||
:<math> | :<math> | ||
| Line 127: | Line 129: | ||
== Other thermodynamic parameters == | == Other thermodynamic parameters == | ||
The extensive volume ''V'' is related to the volume per particle '' | The extensive (i.e., linear in ''n'') volume ''V'' is related to the volume ''v'' per particle ''V''= ''Nv'', where ''N = nN''<sub>A</sub> is the number of particles in the system. As before, the system consists of structureless particles with three translational degrees of freedom only. | ||
We consider the statistical-thermodynamic equation for the [[Helmholtz free energy]] ''A'', | |||
:<math> | :<math> | ||
A = -kT \ln Q.\, | A = -kT \ln Q.\, | ||
| Line 137: | Line 140: | ||
-\frac{n^2 a}{V}</math> | -\frac{n^2 a}{V}</math> | ||
This equation expresses ''A'' in terms of its | This equation expresses ''A'' in terms of its natural variables ''V'' and ''T'', and gives us all thermodynamic information about the system. | ||
For instance, the mechanical equation of state (the van der Waals equation) was already derived above | |||
:<math>p = -\left(\frac{\partial A}{\partial V}\right)_T | :<math>p = -\left(\frac{\partial A}{\partial V}\right)_T | ||
= \frac{NkT}{V-nb}-\frac{n^2 a}{V^2}</math> | = \frac{NkT}{V-nb}-\frac{n^2 a}{V^2}</math> | ||
The | The [[entropy (thermodynamics)|entropy]] (''S'' ) of a van der Waals fluid follows easily from ''A'', it is: | ||
:<math>S = -\left(\frac{\partial A}{\partial T}\right)_V | :<math>S = -\left(\frac{\partial A}{\partial T}\right)_V | ||
| Line 149: | Line 154: | ||
from which we can calculate the [[internal energy]] | from which we can calculate the [[internal energy]] | ||
:<math>U | :<math>U \equiv A+TS = \frac{3}{2}\,NkT-\frac{n^2 a}{V}.</math> | ||
Similar equations can be | Writing ''U'' in terms of its natural variables ''S'' and ''V'' requires elimination of ''T'', that is, it needs an expression for ''T'' as a function of ''S'' and ''V''. This can be obtained without great difficulty from the above expression for ''S''. | ||
Similar equations can be derived from ''A'' for other thermodynamic [[characteristic function (thermodynamics)|characteristic function]]s, but expressing, for instance, the enthalpy ''H'' ≡ ''A'' + ''TS'' + ''pV'' as function of its natural variables ''p'' and ''S'', will require writing ''T'' and ''V'' as explicit functions of ''p'' and ''S'', which yields a complicated expression for ''H''. Also the [[Gibbs free energy]] ''G'' ≡ ''A''+''pV'' will be a complicated function of its natural variables ''T'' and ''p''. | |||
==Reduced form== | ==Reduced form== | ||
| Line 177: | Line 184: | ||
==References== | ==References== | ||
<references /> | <references /> | ||
[[Category:Suggestion Bot Tag]] | |||
Latest revision as of 07:01, 4 November 2024
In physics, chemistry, and chemical engineering, the van der Waals equation is an equation of state for a fluid composed of particles that have a non-zero size and a pairwise attractive interparticle force (such as the van der Waals force). It was derived by Johannes Diderik van der Waals in his doctoral thesis (Leiden 1873)[1] by modification of the ideal gas law. The importance of this work is the recognition that the gas and liquid phase of a substance transform continuously into each other. Above the critical temperature, there is no difference between the gas and liquid phase, which is why it is proper to speak of the equation of a fluid, a generic term for liquid and gas.
Equation
Consider a vessel of volume V filled with n moles of particles (atoms or molecules) of a single compound. Let the pressure be p and the absolute temperature be T, then the van der Waals equation reads
- ,
where
- a is a measure of the strength of attraction between the particles,
- b is the volume excluded from V by one mole of particles,
- R is the gas constant, R = NA k, where k is the Boltzmann constant and NA is Avogadro's constant.
The van der Waals parameter b is proportional to NA times the volume of a single particle—the volume bounded by the atomic radius. In van der Waals' original derivation, given below, b/NA is 4 times the volume of a single particle. Particles are seen as hard spheres. Observe that the pressure p goes to infinity when the container is completely filled, so that there is no void space left for the particles to move. This occurs when V = n b.
Although the parameters a and b are referred to as "the Van der Waals constants", they are not truly constants because they vary from one gas to another; they are, however, independent of P, V, and T. In other words, they are constant for the specific gas being considered.
Illustration
From the empirical critical temperature TC and the empirical critical pressure pC the constants a and b can be obtained by the equations
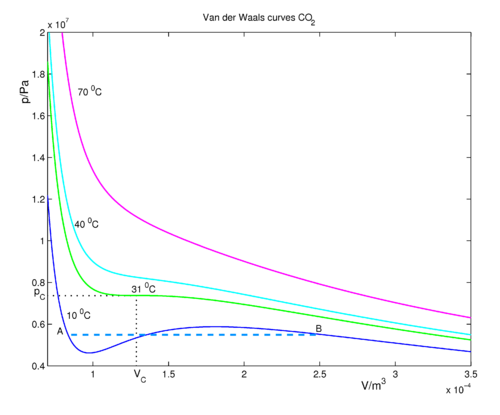
The carbon dioxide molecule (CO2) has[2] pC = 7.37 MPa and TC = 304.1 K = 31 ˚C , which gives a = 0.366 m6 Pa and b = 42.9 10-6 m3. For four different temperatures the van der Waals curves are given in the following figure. The critical pressure is at 73.7 bar = 7370 kPa (is close to 73.7 atm).
For large volumes (on the right-hand side of the figure), the pressures are low and the fluid obeys approximately the ideal gas law pV = nRT for all temperatures shown. If we decrease the volume (go to the left in the figure along an isotherm), the pressure rises. Consider the (blue) isotherm of 10 0C, which is below the critical temperature. Decrease the volume until we reach the point B, where condensation (formation of liquid CO2) starts. At this point the van der Waals curve is no longer physical (excluding the possibility of the occurrence of an oversaturated, metastable gas), because in reality from B to the left (to smaller volume), the pressure stays constant—equal to the vapor pressure of the liquid. The real physical behavior is given by the dashed line of gas-liquid coexistence. The areas, bounded by the 10 °C isotherm, below and above the coexistence line are equal. This is the content of Maxwell's equal-area rule. Decreasing the volume further, we end at point A where all molecules are now in the liquid phase, no molecules are remaining in the gas phase. When the volume is diminished of a vessel that contains only liquid, the pressure rises steeply, because the compressibility of a liquid is considerable smaller than that of a gas.
If one follows the (green) 31 °C curve of critical temperature, one meets a horizontal point of inflection (first and second derivatives of p with respect to V are zero). Note that the figure exhibits two isotherms of temperature higher than the critical temperature; if they are followed, no liquid–gas phase transition will be seen; the higher pressure fluid will resemble a liquid, while at lower pressures the fluid will be more gaslike. At temperatures higher than the critical temperature, no gas–liquid interface occurs.
Although the maxima and minima in the van der Waals curves below the critical point are not physical, the equation for these curves, derived by van der Waals in 1873, was a great scientific achievement. Even today it is not possible to give a single equation that describes correctly the gas–liquid phase transition.
Validity
Above the critical temperature the van der Waals equation is an improvement of the ideal gas law, and for lower temperatures the equation is also qualitatively reasonable for the liquid state and the low-pressure gaseous state. However, the van der Waals model cannot be taken seriously in a quantitative sense; it is only useful for qualitative purposes.[3]
In the first-order phase transition range of (p,V,T) (where the liquid phase and the gas phase are in equilibrium) it does not exhibit the empirical fact that p is constant (equal to the vapor pressure of the liquid) as a function of V for a given temperature.
Derivation
In the usual textbooks one finds two different derivations. One is the conventional derivation that goes back to van der Waals and the other is a statistical mechanics derivation. The latter has as its major advantage that it makes explicit the intermolecular potential, which is out of sight in the first derivation.
Conventional derivation
Consider first one mole of gas which is composed of non-interacting point particles that satisfy the ideal gas law
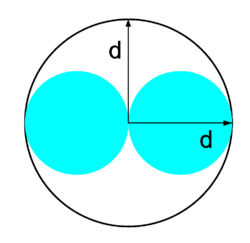
Next assume that all particles are hard spheres of the same finite radius r = d/2 (the van der Waals radius). The effect of the finite volume of the particles is to decrease the available void space in which the particles are free to move. We must replace V by V-b, where b is called the excluded volume. The corrected equation becomes
The excluded volume b is not just equal to NA times the volume occupied by a single particle, but actually 4 NA times that volume. To see this we must realize that two colliding particles are enveloped by a sphere of radius d that is forbidden for the centers of the other particles, see the figure. If the center of a third particle would come into the enveloping sphere, it would mean that the third particle penetrates any of the other two, which, by definition, hard spheres are unable to do. The excluded volume b/NA per particle pair is 4π/3 × d3 = 32π/3 × r3, so that the excluded volume is not 8π/3 × r3 (the sum of volumes of the two particles), but four times as much.[4]
It was a point of concern to van der Waals that the factor 4 yields actually an upper bound; empirical values for b are usually lower. Of course molecules are not infinitely hard, as van der Waals assumed, but are often fairly soft.
Next, we introduce a pairwise attractive force between the particles. Van der Waals assumed that, notwithstanding the existence of this force, the density of the fluid is homogeneous. Furthermore, he assumed that the range of the attractive force is so small that the great majority of the particles do not feel that the container is of finite size. That is, the bulk of the particles do not notice that they have more attracting particles to their right than to their left when they are relatively close to the left-hand wall of the container. The same statement holds with left and right interchanged. Given the homogeneity of the fluid, the bulk of the particles do not experience a net force pulling them to the right or to the left. This is different for the particles in surface layers directly adjacent to the walls. They feel a net force from the bulk particles pulling them into the container, because this force is not compensated by particles between them and the wall (another assumption here is that there is no interaction between walls and particles). This net force decreases the force exerted onto the wall by the particles in the surface layer. The net force on a surface particle, pulling it into the container, is proportional to the number density C ≡ NA/Vm. The number of particles in the surface layers is, again by assuming homogeneity, also proportional to the density. In total, the force on the walls is decreased by a factor proportional to the square of the density and the pressure (force per unit surface) is decreased by
- ,
so that
Upon writing n for the number of moles and nVm = V, the equation obtains the form given above,
It is of some historical interest to point out that van der Waals in his Nobel prize lecture gave credit to Laplace for the argument that pressure is reduced proportional to the square of the density.
Statistical thermodynamics derivation
The canonical partition function Q of an ideal gas consisting of N = nNA identical particles, is
where is the thermal de Broglie wavelength,
with the usual definitions: h is Planck's constant, m the mass of a particle, k Boltzmann's constant and T the absolute temperature. In an ideal gas q is the partition function of a single particle in a vessel of volume V. In order to derive the van der Waals equation, we assume now that each particle moves independently in an average potential field offered by the other particles. The averaging over the particles is easy because we will assume that the particle density of the van der Waals fluid is homogeneous. The interaction between a pair of particles, which are hard spheres, is taken to be
r is the distance between the centers of the spheres and d is the distance where the hard spheres touch each other (twice the van der Waals radius). The depth of the van der Waals well is .
Because the particles are independent the total partition function still factorizes, , but the intermolecular potential necessitates two modifications to q. First, because of the finite size of the particles, not all of V is available, but only , where (excluded volume per particle). Second, we insert a Boltzmann factor to take care of the average intermolecular potential. We divide here the potential by two because this interaction energy is shared between two particles. Thus
The total attraction felt by a single particle is,
where we assumed that in a shell of thickness dr there are N/V 4π r2dr particles. Performing the integral we get
Hence, we obtain by the use of Stirling's approximation,
It is convenient to take T out of Λ and to rewrite this expression as
where Φ is a constant. From statistical thermodynamics we know that
- ,
so that, writing
we get,
Other thermodynamic parameters
The extensive (i.e., linear in n) volume V is related to the volume v per particle V= Nv, where N = nNA is the number of particles in the system. As before, the system consists of structureless particles with three translational degrees of freedom only.
We consider the statistical-thermodynamic equation for the Helmholtz free energy A,
From the equation derived above for lnQ, we find
This equation expresses A in terms of its natural variables V and T, and gives us all thermodynamic information about the system.
For instance, the mechanical equation of state (the van der Waals equation) was already derived above
The entropy (S ) of a van der Waals fluid follows easily from A, it is:
from which we can calculate the internal energy
Writing U in terms of its natural variables S and V requires elimination of T, that is, it needs an expression for T as a function of S and V. This can be obtained without great difficulty from the above expression for S.
Similar equations can be derived from A for other thermodynamic characteristic functions, but expressing, for instance, the enthalpy H ≡ A + TS + pV as function of its natural variables p and S, will require writing T and V as explicit functions of p and S, which yields a complicated expression for H. Also the Gibbs free energy G ≡ A+pV will be a complicated function of its natural variables T and p.
Reduced form
Although the material constants a and b in the van der Waals equation depend on the fluid considered, the equation can be recast into a single form applicable to all fluids.
The critical pressure and critical volume can be computed from the condition that the tangent in the critical point is horizontal and that it is a point of inflection:
This gives
Defining the following reduced variables:
- ,
the van der Waals equation of state given above (with n = 1) can be recast in the following reduced form:
This equation holds for all fluids. Thus, when measured in units of the critical values of various quantities, all fluids obey the same equation of state—the reduced van der Waals equation of state. Van der Waals termed this the principle of corresponding states.
References
- ↑ van der Waals, JD (1873) Over de Continuiteit van den Gas- en Vloeistoftoestand (on the continuity of the gas and liquid state). Ph.D. thesis, Leiden, The Netherlands. [1]
- ↑ J. V. Sengers and J. M. H. Levelt-Sengers, Annual Review of Physical Chemistry, vol. 37, p. 189 (1986)
- ↑ T. L. Hill, Statistical Thermodynamics, Addison-Wesley, Reading (1960), p. 280
- ↑ That the argument leading to this factor is not completely trivial appears from the fact that Maxwell considered it to be erroneous; Maxwell derived a factor 16. See, A. Ya. Kipnis, B. E. Yavelov, and J. S. Rowlinson, Van der Waals and Molecular Science, Oxford University Press, (1996) p. 64















![{\displaystyle \exp[-\phi /(2kT)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e5a94595f750dac5aa4133e29923987e04f1e264)




![{\displaystyle \ln Q=N\left(1+\ln {\Bigg [}{\frac {(V-Nb')\,T^{3/2}}{N\Phi }}{\Bigg ]}\right)+{\frac {N^{2}a'}{VkT}},}](https://wikimedia.org/api/rest_v1/media/math/render/svg/55014f0ac9a0dad91981c210af86a007153200cc)






![{\displaystyle S=-\left({\frac {\partial A}{\partial T}}\right)_{V}=Nk\left[\ln \left({\frac {(V-nb)T^{3/2}}{N\Phi }}\right)+{\frac {5}{2}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/be3fa7b55e9ddcf6a3aecee178267713077872cf)




