Two-port network: Difference between revisions
imported>John R. Brews |
mNo edit summary |
||
| (22 intermediate revisions by 2 users not shown) | |||
| Line 2: | Line 2: | ||
{{TOC|right}} | {{TOC|right}} | ||
{{Image|Two-port with Thevenin driver.PNG|right|350px| Two-port network with symbol definitions. A [[ | {{Image|Two-port with Thevenin driver.PNG|right|350px| Two-port network with symbol definitions. A [[Thévenin's theorem|Thévenin voltage source]] with [[Thévenin's theorem|Thévenin impedance]] ''Z<sub>Th</sub>'' drives {{nowrap|port 1,}} and impedance ''Z<sub>L</sub>'' loads {{nowrap|port 2}}}} | ||
A '''two-port network''' is an [[electrical circuit]] with two ''pairs'' of terminals. As shown in the figure, two terminals constitute a '''port''' ''only'' if they satisfy the essential requirement known as the '''port condition''', namely, the same current must enter and leave a port.<ref name=Gray/><ref name=Jaeger/> | A '''two-port network''' is an [[electrical circuit]] with two ''pairs'' of terminals. As shown in the figure, two terminals constitute a '''port''' ''only'' if they satisfy the essential requirement known as the '''port condition''', namely, the same current must enter and leave a port.<ref name=Gray/><ref name=Jaeger/> | ||
Examples include small-signal models for transistors (such as the [[hybrid-pi model]]), [[electronic filter|filter]]s and [[matching network]]s. The analysis of passive two-port networks is an outgrowth of reciprocity theorems first derived by Lorentz.<ref name=Jasper/> | Examples include small-signal models for transistors (such as the [[hybrid-pi model]]), [[electronic filter|filter]]s and [[matching network]]s. The analysis of passive two-port networks is an outgrowth of reciprocity theorems first derived by Lorentz.<ref name=Jasper/> | ||
A two-port network makes possible the replacement of either a complete circuit or part of it by a "[[black box]]" with | A two-port network makes possible the replacement of either a complete circuit or part of it by a "[[black box]]" with only four distinct parameters, enabling us to separate its behavior from that of its internal constituents, thus simplifying analysis. Any linear circuit with four terminals can be transformed into a two-port network provided that it does not contain an independent source and satisfies the port conditions. | ||
The parameters used to describe a two-port network are the following: z, y, h, g. Each choice corresponds to a different choice for which pair of variables from port 1 and port 2 are chosen to be independent, externally applied sources and which two will be the dependent resultant variables determine by the two-port parameters (see the figure). The port currents and voltages are denoted as follows: | The parameters used to describe a two-port network are the following: z, y, h, g. Each choice corresponds to a different choice for which pair of variables from {{nowrap|port 1}} and {{nowrap|port 2}} are chosen to be independent, externally applied sources and which two will be the dependent resultant variables determine by the two-port parameters (see the figure). The port currents and voltages are denoted as follows: | ||
:< | :''V<sub>1</sub>'' = Port 1 voltage | ||
:< | :''I<sub>1</sub>''  = Port 1 current | ||
:< | :''V<sub>2</sub>'' = Port 2 voltage | ||
:< | :''I<sub>2</sub>''  = Port 2 current | ||
The relations between inputs and outputs usually are expressed in matrix notation. | The relations between inputs and outputs usually are expressed in matrix notation. | ||
| Line 21: | Line 21: | ||
== Impedance parameters (z-parameters)== | == Impedance parameters (z-parameters)== | ||
{{Image|Z-equivalent two-port.PNG|right|350px| Z-equivalent two port showing independent variables ''I<sub>1</sub>'' and ''I<sub>2</sub>''.}} | {{Image|Z-equivalent two-port.PNG|right|350px| Z-equivalent two port showing independent variables ''I<sub>1</sub>'' and ''I<sub>2</sub>''.}} | ||
The figure shows the two-port driven by two external current sources, making the input currents ''I<sub>1</sub>'' and ''I<sub>2</sub>'' the independent variables controlled from outside the two-port. The port voltages are determined in terms of these input currents by the ''z''-parameters defined by: | The figure shows the two-port driven by two external current sources, making the input currents ''I<sub>1</sub>'' and ''I<sub>2</sub>'' the independent variables controlled from outside the two-port. By convention, dependent sources (controlled by the independent sources) are depicted using diamond shapes. The port voltages are determined in terms of these input currents by the ''z''-parameters defined by: | ||
:<math> \left[ \begin{array}{c} V_1 \\ V_2 \end{array} \right] = \left[ \begin{array}{cc} z_{11} & z_{12} \\ z_{21} & z_{22} \end{array} \right] \left[ \begin{array}{c}I_1 \\ I_2 \end{array} \right] </math>. | :<math> \left[ \begin{array}{c} V_1 \\ V_2 \end{array} \right] = \left[ \begin{array}{cc} z_{11} & z_{12} \\ z_{21} & z_{22} \end{array} \right] \left[ \begin{array}{c}I_1 \\ I_2 \end{array} \right] </math>. | ||
| Line 31: | Line 31: | ||
===Example: bipolar [[current mirror]] with emitter degeneration=== | ===Example: bipolar [[current mirror]] with emitter degeneration=== | ||
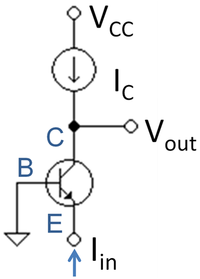
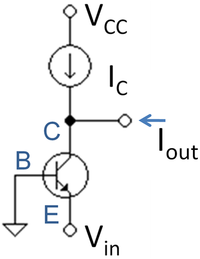
{{Image|Bipolar current mirror with emitter resistors.PNG|left|200px| Bipolar current mirror: ''I<sub>1</sub>'' is the ''reference current'' and ''I<sub>2</sub>'' is the ''output current''.}} | {{Image|Bipolar current mirror with emitter resistors.PNG|left|200px| [[Bipolar transistor]] [[current mirror]]: ''I<sub>1</sub>'' is the ''reference current'' and ''I<sub>2</sub>'' is the ''output current''.}} | ||
{{Image|Small-signal circuit for bipolar mirror.PNG|right|300px| Small-signal circuit for bipolar current mirror: ''I<sub>1</sub>'' is the amplitude of the small-signal ''reference current'' and ''I<sub>2</sub>'' is the amplitude of the small-signal ''output current''.}} | {{Image|Small-signal circuit for bipolar mirror.PNG|right|300px| Small-signal circuit for bipolar current mirror: ''I<sub>1</sub>'' is the amplitude of the small-signal ''reference current'' and ''I<sub>2</sub>'' is the amplitude of the small-signal ''output current''.}} | ||
The figure at left shows a bipolar current mirror with emitter resistors to increase its output resistance.<ref name=feedback/> Transistor ''Q<sub>1</sub>'' is ''diode connected'', which is to say its collector-base voltage is zero. The figure at right shows the small-signal circuit equivalent to the transistor circuit. Transistor ''Q<sub>1</sub>'' is represented by its emitter resistance ''r<sub>E</sub>'' ≈ ''V<sub>T</sub> / I<sub>E</sub>'' (''V<sub>T</sub>'' = thermal voltage, ''I<sub>E</sub>'' = [[Q-point]] emitter current), a simplification made possible because the dependent current source in the hybrid-pi model for ''Q<sub>1</sub>'' draws the same current as a resistor 1 / ''g<sub>m</sub>'' connected across ''r<sub>π</sub>''. The second transistor ''Q<sub>2</sub>'' is represented by its [[hybrid-pi model]]. Table 1 below shows the z-parameter expressions that make the z-equivalent two-port electrically equivalent to the small-signal circuit for the mirror. | The figure at left shows a [[Bipolar transistor|bipolar]] [[current mirror]] with emitter resistors to increase its output resistance.<ref name=feedback/> Transistor ''Q<sub>1</sub>'' is ''diode connected'', which is to say its collector-base voltage is zero. The figure at right shows the small-signal circuit equivalent to the transistor circuit. Transistor ''Q<sub>1</sub>'' is represented by its emitter resistance ''r<sub>E</sub>'' ≈ ''V<sub>T</sub> / I<sub>E</sub>'' (''V<sub>T</sub>'' = thermal voltage, ''I<sub>E</sub>'' = [[Mode (electronics)|Q-point]] emitter current), a simplification made possible because the dependent current source in the hybrid-pi model for ''Q<sub>1</sub>'' draws the same current as a resistor 1 / ''g<sub>m</sub>'' connected across ''r<sub>π</sub>''. The second transistor ''Q<sub>2</sub>'' is represented by its [[hybrid-pi model]]. Table 1 below shows the z-parameter expressions that make the z-equivalent two-port electrically equivalent to the small-signal circuit for the mirror. | ||
{| class="wikitable" style="background:white;text-align:center;margin: 1em auto 1em auto" | {| class="wikitable" style="background:white;text-align:center;margin: 1em auto 1em auto" | ||
!Table 1 !! Expression !! Approximation | !Table 1 !! Expression !! Approximation | ||
| Line 44: | Line 44: | ||
|-valign="center" | |-valign="center" | ||
|<math>Z_{11}= \begin{matrix} \frac{V_{1}}{I_{1}}\end{matrix} \Big|_{I_{2}=0} </math> | |<math>Z_{11}= \begin{matrix} \frac{V_{1}}{I_{1}}\end{matrix} \Big|_{I_{2}=0} </math> | ||
|<math> (r_E + R_E)</math> <math> | |<math> (r_E + R_E)</math> <math>\mathit{\parallel} </math> <math>(r_{ \pi} +R_E) </math> | ||
|<math></math> | |<math></math> | ||
| Line 57: | Line 57: | ||
|<math>R_E</math> <math> \begin{matrix} \frac {r_E+R_E} {r_{ \pi} +2R_E} \end{matrix}</math> | |<math>R_E</math> <math> \begin{matrix} \frac {r_E+R_E} {r_{ \pi} +2R_E} \end{matrix}</math> | ||
|} | |} | ||
In this table, the bipolar transistor ''emitter resistance'' denoted ''r<sub>E</sub>'' is defined as: | |||
:<math>r_E=\frac{r_\pi}{\beta+1} \ , </math> | |||
and the notation '||' indicates the resistance of resistors in parallel: | |||
:<math> R_1\mathit{\parallel}R_2 = \left(\frac{1}{R_1} + \frac{1}{R_2} \right)^{-1} \ . </math> | |||
The negative feedback introduced by resistors ''R<sub>E</sub>'' can be seen in these parameters. For example, when used as an active load in a differential amplifier, ''I<sub>1</sub> ≈ -I<sub>2</sub>'', making the output impedance of the mirror approximately ''R<sub>22</sub> -R<sub>21</sub>'' ≈ 2 β ''r<sub>O</sub>R<sub>E</sub>'' /( ''r<sub>π</sub>+2R<sub>E</sub>'' ) compared to only ''r<sub>O</sub>'' without feedback (that is with ''R<sub>E</sub>'' = 0 Ω) . At the same time, the impedance on the reference side of the mirror is approximately ''R<sub>11</sub> -R<sub>12</sub>'' ≈ <math> \begin{matrix} \frac {r_{\pi}} {r_{\pi}+2R_E} \end{matrix} </math> <math> (r_E+R_E)</math>, only a moderate value, but still larger than ''r<sub>E</sub>'' with no feedback. In the differential amplifier application, a large output resistance increases the difference-mode gain, a good thing, and a small mirror input resistance is desirable to avoid [[Miller effect]]. | The negative feedback introduced by resistors ''R<sub>E</sub>'' can be seen in these parameters. For example, when used as an active load in a differential amplifier, ''I<sub>1</sub> ≈ -I<sub>2</sub>'', making the output impedance of the mirror approximately ''R<sub>22</sub> -R<sub>21</sub>'' ≈ 2 β ''r<sub>O</sub>R<sub>E</sub>'' /( ''r<sub>π</sub>+2R<sub>E</sub>'' ) compared to only ''r<sub>O</sub>'' without feedback (that is with ''R<sub>E</sub>'' = 0 Ω) . At the same time, the impedance on the reference side of the mirror is approximately ''R<sub>11</sub> -R<sub>12</sub>'' ≈ <math> \begin{matrix} \frac {r_{\pi}} {r_{\pi}+2R_E} \end{matrix} </math> <math> (r_E+R_E)</math>, only a moderate value, but still larger than ''r<sub>E</sub>'' with no feedback. In the differential amplifier application, a large output resistance increases the difference-mode gain, a good thing, and a small mirror input resistance is desirable to avoid [[Miller effect]]. | ||
| Line 77: | Line 84: | ||
==Hybrid parameters (h-parameters) == | ==Hybrid parameters (h-parameters) == | ||
{{Image|H-equivalent two-port.PNG|right|350px|H-equivalent two-port showing independent variables ''I<sub>1</sub>'' and ''V<sub>2</sub>''.}} | {{Image|H-equivalent two-port.PNG|right|350px|H-equivalent two-port showing independent variables ''I<sub>1</sub>'' and ''V<sub>2</sub>''.}} | ||
The figure shows the two-port driven by two external sources, a current source at port 1 and a voltage source at port 2, making the input current ''I<sub>1</sub>'' and input voltage ''V<sub>2</sub>'' the independent variables controlled from outside the two-port. The voltage at port 1, ''V<sub>1</sub>'', and the current at port 2, ''I<sub>2</sub>'', are determined in terms of these inputs by the ''h''-parameters defined by: | The figure shows the two-port driven by two external sources, a current source at {{nowrap|port 1}} and a voltage source at {{nowrap|port 2,}} making the input current ''I<sub>1</sub>'' and input voltage ''V<sub>2</sub>'' the independent variables controlled from outside the two-port. The voltage at {{nowrap|port 1,}} ''V<sub>1</sub>'', and the current at {{nowrap|port 2,}} ''I<sub>2</sub>'', are determined in terms of these inputs by the ''h''-parameters defined by: | ||
:<math> {V_1 \choose I_2} = \begin{pmatrix} h_{11} & h_{12} \\ h_{21} & h_{22} \end{pmatrix}{I_1 \choose V_2} </math>. | :<math> {V_1 \choose I_2} = \begin{pmatrix} h_{11} & h_{12} \\ h_{21} & h_{22} \end{pmatrix}{I_1 \choose V_2} </math>. | ||
| Line 86: | Line 93: | ||
:<math>h_{21} = {I_2 \over I_1 } \bigg|_{V_2 = 0} \qquad h_{22} = {I_2 \over V_2 } \bigg|_{I_1 = 0}</math> | :<math>h_{21} = {I_2 \over I_1 } \bigg|_{V_2 = 0} \qquad h_{22} = {I_2 \over V_2 } \bigg|_{I_1 = 0}</math> | ||
Often this circuit is selected when a current amplifier is described, because the port 1 input is the independent input current and port 2 output is the dependent current. | Often this circuit is selected when a current amplifier is described, because the {{nowrap|port 1}} input is the independent input current and {{nowrap|port 2}} output is the dependent current. | ||
Notice that off-diagonal h-parameters are dimensionless, while the series-connected diagonal element has dimensions of ohms, while the shunt-connected diagonal element has dimensions of siemens. | Notice that off-diagonal h-parameters are dimensionless, while the series-connected diagonal element has dimensions of ohms, while the shunt-connected diagonal element has dimensions of siemens. | ||
===Example: common base amplifier=== | ===Example: common-base amplifier=== | ||
{{Image|Common base with current drive.PNG|left|200px|Common base circuit with active load and current | {{Image|Common base with current drive.PNG|left|200px|Common-base amplifier circuit with active load and signal current injected into emitter.}} | ||
{{Image|Common base with current driver.PNG|right|300px|Common-base amplifier with AC current source ''I<sub>1</sub>'' as signal input and unspecified load supporting voltage ''V<sub>2</sub>'' and a dependent current ''I<sub>2</sub>''.}} | {{Image|Common base with current driver.PNG|right|300px|Common-base amplifier with AC current source ''I<sub>1</sub>'' as signal input and unspecified load supporting voltage ''V<sub>2</sub>'' and a dependent current ''I<sub>2</sub>''.}} | ||
The common base amplifier is shown at left. The current source in the collector lead is an ideal DC source, and as such will not allow passage of any varying signal through itself. Its purpose in the circuit is to provide a DC bias current that sets the operating point (the quiescent transistor state about which the transistor varies in processing the signal). A signal ''current'' is applied to the emitter, and an output ''voltage'' is required at the collector. The output node is shown as an open circuit (no load) at the left, but to make use of the amplifier, a load can be attached, which then will draw a signal current from the collector node. | The [[Bipolar transistor|bipolar]] common-base amplifier is shown at left. The current source in the collector lead is an ideal DC source, and as such will not allow passage of any varying signal through itself. Its purpose in the circuit is to provide a DC bias current that sets the operating point (the quiescent transistor state about which the transistor varies in processing the signal; see the article [[Mode (electronics)]].). A signal ''current'' is applied to the emitter, and an output ''voltage'' is required at the collector. The output node is shown as an open circuit (no load) at the left, but to make use of the amplifier, a load can be attached, which then will draw a signal current from the collector node. | ||
The small-signal circuit at the right assumes the voltage at the collector is a specified variable, and the output current is to be determined. The input current at the emitter also is a specified variable, and the signal voltage ''V<sub>E</sub>'' developed at the emitter is a dependent variable. | The small-signal circuit at the right assumes the voltage at the collector is a specified variable, and the output current is to be determined. The input current at the emitter also is a specified variable, and the signal voltage ''V<sub>E</sub>'' developed at the emitter is a dependent variable. | ||
| Line 109: | Line 116: | ||
|-valign="center" | |-valign="center" | ||
|<math>h_{11}= \begin{matrix} \frac{V_{1}}{I_{1}}\end{matrix} \Big|_{V_{2}=0} </math> | |<math>h_{11}= \begin{matrix} \frac{V_{1}}{I_{1}}\end{matrix} \Big|_{V_{2}=0} </math> | ||
|<math> r_E | |<math> r_E\mathit{\parallel}r_O </math> | ||
|<math>r_E</math> | |<math>r_E</math> | ||
| Line 122: | Line 129: | ||
|<math>\ \begin{matrix} \frac {r_E} {r_O} \end{matrix} \ </math> << 1 | |<math>\ \begin{matrix} \frac {r_E} {r_O} \end{matrix} \ </math> << 1 | ||
|} | |} | ||
The transistor β is related to the transistor transconductance ''g<sub>m</sub>'' as: | |||
:<math> \beta=g_m r_\pi \ . </math> | |||
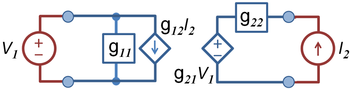
==Inverse hybrid parameters (g-parameters)== | ==Inverse hybrid parameters (g-parameters)== | ||
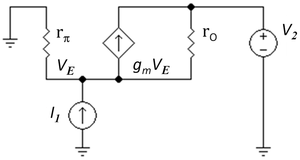
{{Image|G-equivalent two-port.PNG|right|350px| G-equivalent two-port showing independent variables ''V<sub>1</sub>'' and ''I<sub>2</sub>''.}} | {{Image|G-equivalent two-port.PNG|right|350px| G-equivalent two-port showing independent variables ''V<sub>1</sub>'' and ''I<sub>2</sub>''.}} | ||
The figure shows the two-port driven by two external sources, a voltage source at port 1 and a current source at port 2, making the input voltage ''V<sub>1</sub>'' and input current ''I<sub>2</sub>'' the independent variables controlled from outside the two-port. The current at port 1, ''I<sub>1</sub>'', and the voltage at port 2, ''V<sub>2</sub>'', are determined in terms of these inputs by the ''g''-parameters defined by: | The figure shows the two-port driven by two external sources, a voltage source at {{nowrap|port 1}} and a current source at {{nowrap|port 2,}} making the input voltage ''V<sub>1</sub>'' and input current ''I<sub>2</sub>'' the independent variables controlled from outside the two-port. The current at {{nowrap|port 1,}} ''I<sub>1</sub>'', and the voltage at {{nowrap|port 2,}} ''V<sub>2</sub>'', are determined in terms of these inputs by the ''g''-parameters defined by: | ||
:<math> {I_1 \choose V_2} = \begin{pmatrix} g_{11} & g_{12} \\ g_{21} & g_{22} \end{pmatrix}{V_1 \choose I_2} </math>. | :<math> {I_1 \choose V_2} = \begin{pmatrix} g_{11} & g_{12} \\ g_{21} & g_{22} \end{pmatrix}{V_1 \choose I_2} </math>. | ||
| Line 136: | Line 146: | ||
Often this circuit is selected to describe a voltage amplifier, as the port 1 input is an independent voltage, and the port 2 output is a dependent voltage. Notice that off-diagonal g-parameters are dimensionless, while the series-connected diagonal element has dimensions of ohms, while the shunt-connected diagonal element has dimensions of siemens. | Often this circuit is selected to describe a voltage amplifier, as the {{nowrap|port 1}} input is an independent voltage, and the {{nowrap|port 2}} output is a dependent voltage. Notice that off-diagonal g-parameters are dimensionless, while the series-connected diagonal element has dimensions of ohms, while the shunt-connected diagonal element has dimensions of siemens. | ||
===Example: common base amplifier=== | ===Example: common-base amplifier=== | ||
{{Image|Common base with voltage drive.PNG|left|200px|Bipolar transistor | {{Image|Common base with voltage drive.PNG|left|200px|[[Bipolar transistor]] common-base amplifier. The base is grounded and a signal voltage applied to emitter.}} | ||
{{Image|Small-signal common base circuit.PNG|right|300px|Common-base amplifier with AC voltage source ''V<sub>1</sub>'' as signal input and unspecified load delivering current ''I<sub>2</sub>'' at a dependent voltage ''V<sub>2</sub>''.}} | {{Image|Small-signal common base circuit.PNG|right|300px|Common-base amplifier with AC voltage source ''V<sub>1</sub>'' as signal input and unspecified load delivering current ''I<sub>2</sub>'' at a dependent voltage ''V<sub>2</sub>''.}} | ||
The common base amplifier is shown again at the left. This time, a signal ''voltage'' is applied to the emitter, and an output ''current'' is taken from the collector. | The common-base amplifier is shown again at the left. This time, a signal ''voltage'' is applied to the emitter, and an output ''current'' is taken from the collector. | ||
The small-signal circuit at the right assumes the current at the collector is a specified variable, and the output voltage is to be determined. The input voltage at the emitter also is a specified variable, and the signal current driven into the emitter is a dependent variable. | The small-signal circuit at the right assumes the current at the collector is a specified variable, and the output voltage is to be determined. The input voltage at the emitter also is a specified variable, and the signal current driven into the emitter is a dependent variable. | ||
| Line 169: | Line 179: | ||
==References== | ==References== | ||
{{reflist|refs= | {{reflist|refs= | ||
<!-- | |||
*************** | |||
NOTE: Footnotes in this article are formatted using the [[CZ:List-defined references]] methodology for reducing clutter in the edit window. | |||
NOTE: In alphabetical order by name to make locating these sources easier when editing. | |||
**************** | |||
--> | |||
<ref name=feedback> | |||
The emitter-leg resistors counteract any current increase by decreasing the transistor ''V<sub>BE</sub>''. That is, the resistors ''R<sub>E</sub>'' cause negative feedback that opposes change in current. In particular, any change in output voltage results in less change in current than without this feedback, which means the output resistance of the mirror has increased. | |||
</ref> | |||
<ref name=Gray> | <ref name=Gray> | ||
| Line 174: | Line 198: | ||
|author=P.R. Gray, P.J. Hurst, S.H. Lewis, and R.G. Meyer | |author=P.R. Gray, P.J. Hurst, S.H. Lewis, and R.G. Meyer | ||
|title=Analysis and Design of Analog Integrated Circuits | |title=Analysis and Design of Analog Integrated Circuits | ||
|year= | |year= 2009 | ||
|edition= | |edition=Fifth Edition | ||
|publisher=Wiley | |publisher=Wiley | ||
|location=New York | |location=New York | ||
|isbn= | |isbn=978-0-470-24599-6 | ||
| | |chapter=§3.3: Basic single-transistor amplifier stages | ||
|url=http:// | |pages=pp. 173 ''ff'' | ||
|url=http://www.amazon.com/gp/reader/0470245999/ref=sib_dp_pt#reader-link}} | |||
</ref> | </ref> | ||
| Line 199: | Line 224: | ||
See review by {{cite web |url=http://www.ieee.org/organizations/pubs/newsletters/emcs/summer03/jasper.pdf |author= Jasper J. Goedbloed |title=Reciprocity and EMC measurements |work=Presentation at 2003 EMC Zurich Symposium ''and as'' IEEE EMC EMCS Newsletter |year=2003 |pages=pp.93-104 }}</ref> | See review by {{cite web |url=http://www.ieee.org/organizations/pubs/newsletters/emcs/summer03/jasper.pdf |author= Jasper J. Goedbloed |title=Reciprocity and EMC measurements |work=Presentation at 2003 EMC Zurich Symposium ''and as'' IEEE EMC EMCS Newsletter |year=2003 |pages=pp.93-104 }}</ref> | ||
</ref> | </ref> | ||
<ref name=Pozar>{{cite book | <ref name=Pozar>{{cite book | ||
| Line 218: | Line 237: | ||
</ref> | </ref> | ||
}} | |||
[[Category:Suggestion Bot Tag]] | |||
Latest revision as of 11:00, 31 October 2024
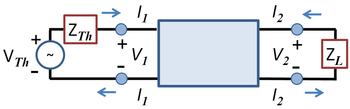
Two-port network with symbol definitions. A Thévenin voltage source with Thévenin impedance ZTh drives port 1, and impedance ZL loads port 2
A two-port network is an electrical circuit with two pairs of terminals. As shown in the figure, two terminals constitute a port only if they satisfy the essential requirement known as the port condition, namely, the same current must enter and leave a port.[1][2] Examples include small-signal models for transistors (such as the hybrid-pi model), filters and matching networks. The analysis of passive two-port networks is an outgrowth of reciprocity theorems first derived by Lorentz.[3]
A two-port network makes possible the replacement of either a complete circuit or part of it by a "black box" with only four distinct parameters, enabling us to separate its behavior from that of its internal constituents, thus simplifying analysis. Any linear circuit with four terminals can be transformed into a two-port network provided that it does not contain an independent source and satisfies the port conditions.
The parameters used to describe a two-port network are the following: z, y, h, g. Each choice corresponds to a different choice for which pair of variables from port 1 and port 2 are chosen to be independent, externally applied sources and which two will be the dependent resultant variables determine by the two-port parameters (see the figure). The port currents and voltages are denoted as follows:
- V1 = Port 1 voltage
- I1 = Port 1 current
- V2 = Port 2 voltage
- I2 = Port 2 current
The relations between inputs and outputs usually are expressed in matrix notation.
These variables are most useful at low to moderate frequencies. At high frequencies (for example, microwave frequencies) power and energy are more useful variables, and the two-port approach based on current and voltages that is discussed here is replaced by an approach based upon scattering parameters.[4]
Though some authors use the terms two-port network and four-terminal network interchangeably, the latter represents a more general concept. Not all four-terminal networks are two-port networks. A pair of terminals can be called a port only if the current entering one is equal to the current leaving the other (the port condition). Only those four-terminal networks in which the four terminals can be paired into two ports can be called two-ports.[1][2]
Impedance parameters (z-parameters)
The figure shows the two-port driven by two external current sources, making the input currents I1 and I2 the independent variables controlled from outside the two-port. By convention, dependent sources (controlled by the independent sources) are depicted using diamond shapes. The port voltages are determined in terms of these input currents by the z-parameters defined by:
- .
Notice that all the series connected elements represented by z-parameters have dimensions of ohms, as do the dependent source parameters.
Example: bipolar current mirror with emitter degeneration

Bipolar transistor current mirror: I1 is the reference current and I2 is the output current.
The figure at left shows a bipolar current mirror with emitter resistors to increase its output resistance.[5] Transistor Q1 is diode connected, which is to say its collector-base voltage is zero. The figure at right shows the small-signal circuit equivalent to the transistor circuit. Transistor Q1 is represented by its emitter resistance rE ≈ VT / IE (VT = thermal voltage, IE = Q-point emitter current), a simplification made possible because the dependent current source in the hybrid-pi model for Q1 draws the same current as a resistor 1 / gm connected across rπ. The second transistor Q2 is represented by its hybrid-pi model. Table 1 below shows the z-parameter expressions that make the z-equivalent two-port electrically equivalent to the small-signal circuit for the mirror.
| Table 1 | Expression | Approximation |
|---|---|---|
In this table, the bipolar transistor emitter resistance denoted rE is defined as:
and the notation '||' indicates the resistance of resistors in parallel:
The negative feedback introduced by resistors RE can be seen in these parameters. For example, when used as an active load in a differential amplifier, I1 ≈ -I2, making the output impedance of the mirror approximately R22 -R21 ≈ 2 β rORE /( rπ+2RE ) compared to only rO without feedback (that is with RE = 0 Ω) . At the same time, the impedance on the reference side of the mirror is approximately R11 -R12 ≈ , only a moderate value, but still larger than rE with no feedback. In the differential amplifier application, a large output resistance increases the difference-mode gain, a good thing, and a small mirror input resistance is desirable to avoid Miller effect.
Admittance parameters (y-parameters)
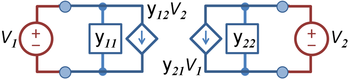
The figure shows the two-port driven by two external voltage sources, making the input voltages V1 and V2 the independent variables controlled from outside the two-port. The port currents are determined in terms of these input voltages by the y-parameters defined by:
- .
where
The network is said to be reciprocal if . Notice that all the shunt-connected elements are represented by y-parameters with dimensions of siemens, as are the dependent source parameters.
Hybrid parameters (h-parameters)
The figure shows the two-port driven by two external sources, a current source at port 1 and a voltage source at port 2, making the input current I1 and input voltage V2 the independent variables controlled from outside the two-port. The voltage at port 1, V1, and the current at port 2, I2, are determined in terms of these inputs by the h-parameters defined by:
- .
where
Often this circuit is selected when a current amplifier is described, because the port 1 input is the independent input current and port 2 output is the dependent current.
Notice that off-diagonal h-parameters are dimensionless, while the series-connected diagonal element has dimensions of ohms, while the shunt-connected diagonal element has dimensions of siemens.
Example: common-base amplifier
The bipolar common-base amplifier is shown at left. The current source in the collector lead is an ideal DC source, and as such will not allow passage of any varying signal through itself. Its purpose in the circuit is to provide a DC bias current that sets the operating point (the quiescent transistor state about which the transistor varies in processing the signal; see the article Mode (electronics).). A signal current is applied to the emitter, and an output voltage is required at the collector. The output node is shown as an open circuit (no load) at the left, but to make use of the amplifier, a load can be attached, which then will draw a signal current from the collector node.
The small-signal circuit at the right assumes the voltage at the collector is a specified variable, and the output current is to be determined. The input current at the emitter also is a specified variable, and the signal voltage VE developed at the emitter is a dependent variable.
Tabulated formulas in Table 2 make the h-equivalent circuit of the transistor agree with the small-signal low-frequency circuit found using the transistor hybrid-pi model at the right. Notation: rπ = base resistance of transistor, rO = output resistance, and gm = transconductance. The negative sign for h21 reflects the convention that I1, I2 are positive when directed into the two-port. Parameter h21 represents the current gain of the circuit viewed as a current amplifier. A non-zero value for h12 means the output voltage affects the input voltage, that is, this amplifier is bilateral. If h12 = 0, the amplifier is unilateral.
| Table 2 | Expression | Approximation |
|---|---|---|
| << 1 |
The transistor β is related to the transistor transconductance gm as:
Inverse hybrid parameters (g-parameters)
The figure shows the two-port driven by two external sources, a voltage source at port 1 and a current source at port 2, making the input voltage V1 and input current I2 the independent variables controlled from outside the two-port. The current at port 1, I1, and the voltage at port 2, V2, are determined in terms of these inputs by the g-parameters defined by:
- .
where
Often this circuit is selected to describe a voltage amplifier, as the port 1 input is an independent voltage, and the port 2 output is a dependent voltage. Notice that off-diagonal g-parameters are dimensionless, while the series-connected diagonal element has dimensions of ohms, while the shunt-connected diagonal element has dimensions of siemens.
Example: common-base amplifier
Bipolar transistor common-base amplifier. The base is grounded and a signal voltage applied to emitter.
The common-base amplifier is shown again at the left. This time, a signal voltage is applied to the emitter, and an output current is taken from the collector.
The small-signal circuit at the right assumes the current at the collector is a specified variable, and the output voltage is to be determined. The input voltage at the emitter also is a specified variable, and the signal current driven into the emitter is a dependent variable.
The tabulated formulas in Table 3 make the g-equivalent two-port for the amplifier agree with the small-signal circuit found using the small-signal low-frequency hybrid-pi model for the transistor. Notation: rπ = base resistance of transistor, rO = output resistance, and gm = transconductance. Parameter g21 represents the voltage gain of this circuit viewed as a voltage amplifier. The negative sign for g12 reflects the convention that I1, I2 are positive when directed into the two-port. A non-zero value for g12 means the output current affects the input current, that is, this amplifier is bilateral. If g12 = 0, the amplifier is unilateral.
| Table 3 | Expression |
|---|---|
References
- ↑ 1.0 1.1 P.R. Gray, P.J. Hurst, S.H. Lewis, and R.G. Meyer (2009). “§3.3: Basic single-transistor amplifier stages”, Analysis and Design of Analog Integrated Circuits, Fifth Edition. New York: Wiley, pp. 173 ff. ISBN 978-0-470-24599-6.
- ↑ 2.0 2.1 R. C. Jaeger and T. N. Blalock (2006). Microelectronic Circuit Design, Third Edition. Boston: McGraw-Hill, §10.5 §13.5 §13.8. ISBN 9780073191638.
- ↑ See review by Jasper J. Goedbloed (2003). Reciprocity and EMC measurements. Presentation at 2003 EMC Zurich Symposium and as IEEE EMC EMCS Newsletter pp.93-104.
- ↑ David M. Pozar (2005). “Chapter 4: Microwave network analysis”, Microwave Engineering, 3rd Edition. John Wiley & Sons, pp. 161-221. ISBN 047164451X (Softcover).
- ↑ The emitter-leg resistors counteract any current increase by decreasing the transistor VBE. That is, the resistors RE cause negative feedback that opposes change in current. In particular, any change in output voltage results in less change in current than without this feedback, which means the output resistance of the mirror has increased.

![{\displaystyle \left[{\begin{array}{c}V_{1}\\V_{2}\end{array}}\right]=\left[{\begin{array}{cc}z_{11}&z_{12}\\z_{21}&z_{22}\end{array}}\right]\left[{\begin{array}{c}I_{1}\\I_{2}\end{array}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7645f82f97b057892f6d77d80bbf01b985aaae0f)

























![{\displaystyle \left[{\begin{array}{c}I_{1}\\I_{2}\end{array}}\right]=\left[{\begin{array}{cc}y_{11}&y_{12}\\y_{21}&y_{22}\end{array}}\right]\left[{\begin{array}{c}V_{1}\\V_{2}\end{array}}\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1f0a34623af2771a0510c92e6a21ac4e6de760aa)